Why Trisecting the Angle is Impossible

Suppose that two lines L₁ and L₂ meet at point P and that the angle θ between the lines is arbitrary. One of the elementary problems in classical geometry is to bisect the angle, that is, to construct a line through P which makes an angle of θ/2 with L₁ and L₂ using only a compass (a tool which can draw a perfect circle of any radius centered at a point) and an unmarked straightedge (a tool which can draw a perfectly straight line through any two points but which cannot be used as a ruler to measure lengths).

To do this construction, start by drawing a circle centered at P, which intersects L₁ and L₂ at A and B, respectively, where θ is the angle between them. Since A and B are points on the same circle centered at P, |PA|=|PB|, and so ΔAPB is isosceles. Therefore if C is the midpoint of the segment AB, then the line segment PC separates ΔAPB into two identical right triangles ΔPCA and ΔPCB, so ∠APC=∠BPC. But ∠APC+∠BPC=θ, so it follows that ∠APC=∠BPC=θ/2, and this completes the construction since PC bisects the angle ∠APC.

This construction is still valid in the cases where θ=0° or 180°, since then ΔAPB is reduced to a straight line segment, which can be thought of as a degenerate isosceles triangle.

It’s natural to ask whether it’s possible to divide an angle into an arbitrary number of equal parts. For example, is it possible to trisect the angle, that is, given a pair of lines with arbitrary angle θ between them, to find a line that makes an angle of θ/3 with either of the lines? This problem, along with the problems of squaring the circle and doubling the cube, would bedevil mathematicians for over 2000 years: why is it so easy to bisect an arbitrary angle, but seemingly insurmountably difficult to trisect an arbitrary angle? This problem was finally solved in 1837 by Pierre Wantzel: the reason that no one has ever managed to trisect the angle with only compass and straightedge is that it is impossible to do so. Now we’re going to look at why.

Problem statement

We should start by clarifying what “trisecting the angle” means. What this means is to find a procedure that, given an arbitrary angle θ, can construct an angle θ/3 in a finite number of steps using only a compass and an unmarked straightedge, or to show that no such procedure exists. Although we will see that the answer is that no procedure to trisect the angle exists which uses only a compass and an unmarked straightedge, it is possible to trisect an angle by other methods.

For example, we could just use trigonometry. Let P be at the origin of a coordinate system and let L₁ coincide with the x-axis. Let Q be the point (arccos(θ/3), arcsin(θ/3)), then the line segment PQ trisects the angle between L₁ and L₂.

If the trigonometric approach feels a little synthetic and boring to you, then don’t worry. There are plenty of constructions that are more in line with the spirit of ancient Greek geometry. For example, there is the solution by Archimedes, which uses a compass and a straightedge with two marks on it (called a neusis ruler). There are also constructions using more elaborate mechanical tools. It is also possible to trisect the angle with origami, as demonstrated by Numberphile:

Some angles can be trisected by compass and straightedge. For example, a 180° angle can be trisected by constructing a pair of equilateral triangles. A 90° angle can be trisected by constructing a 30° angle on each of the two lines. In general, it turns out that an angle whose measure is 2π/N can be trisected if and only if N is not divisible by three.

So it is possible to trisect any angle with other tools, but why are there angles that cannot be trisected with compass and straightedge? Let’s start by asking what we would need to do in order trisect an angle of, say, 60°.

Let L₁ coincide with the x-axis and let L₂ meet L₁ at the origin at a 60° angle. Our goal is to construct a line that makes a 20-degree angle with the x-axis. This is equivalent to constructing a point whose coordinates are x=cos(20°) and y=sin(20°). If it is possible to construct this point, then it must also be possible to construct the points (cos(20°),0) and (0,sin(20°)).

Consider the triple-angle formula:

Set θ=60° and x=cos(20°). Note that cos(60°)=1/2. Then the x-coordinate of the trisection point satisfies the following polynomial equation:

In the derivation follows, it turns out that this polynomial will be somewhat inconvenient to work with. We can put it into a more convenient form by noting that the point (x,0) is constructible by compass and straightedge if and only if (2x,0) is constructible by compass and straightedge. So replace x with 2x in the above polynomial to obtain what is called the trisection equation for the 60° angle:

So is it possible to construct a point (x,0) where x satisfies the trisection equation?

Constructible numbers

Suppose that we are given a line segment P₀P₁. Then a real number r is said to be constructible if and only if it is possible to construct a line segment of length |r| using only a compass and an unmarked straightedge in a finite number of steps. A constructible point is a point whose coordinates (a,b) are constructible numbers.

The number 0 is constructible since it is the length of the “segment” from P₀ to itself. Since we assume that the line segment P₀P₁ exists, and every line segment has a length, we can define a system of length units in which |P₀P₁|=1, so the number 1 is constructible. Let L be the infinite line passing through segment P₀P₁, an draw a circle centered at P₁ which passes through P₀, and let P₂ be the other point where the circle intersects L. Then |P₀P₂|=2 because P₁ is the midpoint of P₀P₂. Then draw a circle centered at P₂ which passes through P₁ and intersects L again at P₃, and then|P₀P₃|=3. Continuing in this way, we construct all of the natural numbers.

Since 0 and all of the positive integers are constructible, and since if r is a constructible number than -r is also constructible, this means that all integers are constructible.

Our goal now is to show that all rational numbers are constructible. Our approach will be to show that the product and quotient of two constructible numbers is constructible.

Suppose that a, b, and x are all constructible. Then the points (x,0) and (a,b) are constructible, as are the points (0,0) and (1,0):

If line L intersects line K at a point, then constructing a line parallel to L that passes through any other point of K is an elementary geometric construction. So draw a line through (x,0) that is parallel to the segment through (1,0) and (a,b) and which intersects the extension of the line segment through (0,0) and (a,b) at point P:

The triangle with vertices at (0,0), (1,0), and (a,b) is similar to the triangle with vertices at (0,0), (x,0), and P. Therefore the lengths of corresponding sides are all in the same ratio. Since the lengths of the sides that lie on the x-axis are in the ratio x:1, the length of the line segment from (0,0) to P is x times the length of the line segment from (0,0) to (a,b), so the coordinates of P are (xa,xb). This means that given constructible numbers a and x, the product ax can also be constructed, so the product of two constructible numbers is constructible.

The argument that the quotient of two constructible numbers is constructible is almost the same. Start with the following:

Through the point (1,0), draw a line parallel to the line through (x,0) and (a,b), which intersects the line from (0,0) to (a,b) at P:

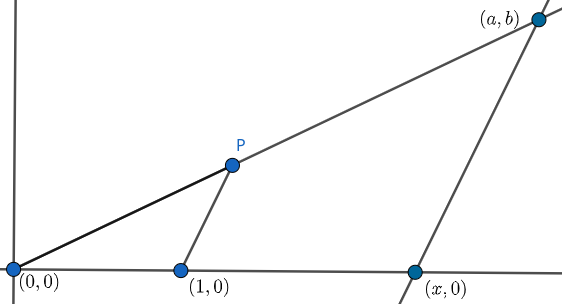
The two triangles are similar and as before the lengths of their corresponding sides are in the ratio x:1, so the coordinates of P are (a/x,b/x). This means that given constructible numbers a and x, it is possible to construct the quotient a/x (assuming, of course, that x is nonzero).

Sums and differences of constructible numbers are also constructible, and the proof is very simple. If a and b are constructible numbers, then the points (a,0) and (b,0) are constructible. The difference is the distance between (a,0) and (b,0), and the sum is the distance between (a,0) and (-b,0).

Since all integers are constructible, and quotients of constructible numbers are constructible, and the rational numbers are by definition all possible quotients of integers, it follows that all rational numbers are constructible.

What about square roots of positive constructible numbers? These are also constructible. Let p be a constructible number and draw a circle of diameter p+1 centered at point M on line L which intersects L at A and B. Choose point D on L so that |DB|=1 and |AD|=p, and erect a perpendicular at D, which intersects the circle at C:

Then by the angle bisector theorem, the length of CD is the square root of p, so square roots of constructible numbers are constructible.

So the rational operations of adding, multiplying, subtracting, and dividing two constructible numbers will always produce a constructible number, and the irrational operation of taking the square root of a constructible number will give a constructible number. Expressions produced by performing these operations on constructible numbers are called quadratic irrationalities. For constructible numbers a, b, and c, the following are some examples of quadratic irrationalities:

We are allowed to take fourth, eighth, sixteenth, etc, roots because if a is constructible then so is √a, and therefore so is √(√a), and so on.

The order of a quadratic irrationality is the maximum depth of all of the nested square roots. A rational number is a quadratic irrationality of order zero. Assuming that a, b, and c are rational, in (1) the order is 1, in (2) the order is 3, in (3) the order is 2 (two square root operations to product a fourth root), and in (4) the order is 6 (the order of the irrationality in the denominator is 2, and then four repeated square root operations to get the eighth root).

A general quadratic irrationality can be written in the form X+Y√Z where X, Y, and Z are quadratic irrationalities and √Z has a higher order than either of X and Y.

Now we want to prove that a constructible number is either rational or a quadratic irrationality of other constructible numbers. This is equivalent to saying that no operations on constructible numbers between addition, multiplication, subtraction, division, and square roots will necessarily produce constructible numbers.

Let S be a set of points with rational coordinates, which are guaranteed to be constructible since we know that the rational numbers are constructible. In compass and straightedge constructions, we are allowed to construct new points only by the following operations:

• Compass operation: Given points A and B in S, draw a circle centered at A which passes through B.
• Straightedge operation: Draw a line through any two points in S.

A new point constructed in this way must be one of the following:

• The point of intersection between two lines drawn by straightedge operations on S.
• A point of intersection between two circles drawn by compass operations on S.
• A point of intersection between a line drawn by a straightedge operation on S and a circle drawn by a compass operation on S.

Let A=(a₁,a₂), B=(b₁,b₂), C=(c₁,c₂), and D=(d₁,d₂) be points in S. Let’s consider the points that can be constructed in a single step from A, B, C, and D.

Let K be the line through A and B and let L be the line through C and D. The equations for these two lines are:

If (x,y) is the point of intersection of these two lines, then x satisfies the following linear equation, and y is obtained by substituting the result back into the equation for K or L:

This is a linear equation and the coefficients are all rational, so x is a rational number and therefore so is y. Therefore x and y are 0th order quadratic irrationalities of rational numbers.

Now suppose that line K intersects with the circle centered at C and passing through D. Then x and y satisfy this system of two equations:

For clarity, rewrite the first equation as y=ux+v, where u and v are rational, and substitute this into the second equation to obtain a quadratic polynomial for x:

These coefficients are all rational so write the polynomial equation for x as Ax²+Bx+C=0 where A, B, and C are rational numbers. Then by the quadratic formula, the solutions for x are:

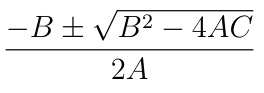
Since A, B, and C are rational, this is a quadratic irrationality of order one.

The case of two intersecting circles reduces to the case of a line intersecting a circle because the points of intersection lie on their shared chord if they intersect in two points or their shared tangent line if they intersect in only one point. The coordinates of the resulting points will therefore also be quadratic irrationalities of order one.

Now let A, B, C, and D be points in S, except this time assume that instead of being rational their coordinates are quadratic irrationalities of arbitrary order. Then by the same reasoning that we just went through, the coordinates of points that can be constructed in a single step from A, B, C, and D are themselves quadratic irrationalities of the coordinates of A, B, C, and D, and therefore ultimately quadratic irrationalities of rational numbers.

It follows that all constructible numbers are quadratic irrationalities of rational numbers. Therefore a number is constructible if and only if it is a quadratic irrationality of rational numbers, so no operations on constructible numbers besides addition, subtraction, multiplication, division, and square roots can produce new constructible numbers.

Proof of impossibility

As we just saw, a number x is constructible only if x may be expressed as a quadratic irrationality of rational numbers. We will show that trisecting a 20° is impossible by showing that none of the solutions of the trisection equation x³-3x-1=0 are quadratic irrationalities.

Suppose that a, b, and c are solutions of the trisection equation. Then we can write the equation as (x-a)(x-b)(x-c)=0, and this can be expanded as:

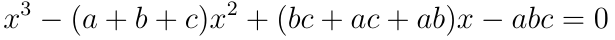
Then a+b+c=0 since the coefficient of x² in the trisection equation is zero. We will need this shortly.

First I will show that the trisection equation does not have any rational roots. Let p and q be integers with no prime factors and common so that p/q is a rational number expressed in lowest terms. If p/q is a solution of the trisection equation then:

Since p and q are both integers, the left-hand side of the second equation is an integer. But since q and p have no prime factors in common, q/p is not an integer, so the right-hand side is not an integer. So if the trisection equation had a rational solution then there would exist some integer that is equal to a non-integer, which is impossible, so the trisection equation has no rational solutions.

Now suppose that A+B√C, with B not equal to zero, is a solution of the trisection equation where A, B, and C are quadratic irrationalities and the order of √C is higher than the order of both A and B so that the order of A+B√C is the order of √C. Plug A+B√C into the equation and expand into the following form:

This can be true only if:

This is why we need to assume that B is not zero, since then the first equation reduces to A³-3A-1=0, which is just the trisection equation for A.

Now let’s see what happens if we replace B with -B:

So A-B√C is also a solution of the trisection equation. Since the roots have to add up to zero, this means that:

And this in turn implies that c=-2A, and A cannot be rational since the equation has no rational solutions, so c must be a quadratic irrationality of order lower than A+B√C and which can be written in the form c=D+E√F where D, E, and F are quadratic irrationalities and the order of √F (which is the order of A) is greater than D and E. But if D+E√F is a root, then so is -2D by the same reasoning as above. Since D cannot be rational, -2D must be a quadratic irrationality of the form G+H√I where G, H, and I are quadratic irrationalities and the order of √I, which is equal to the order of D, is greater than the orders of G and H. Then we would find that -2G is a solution.

So A+B√C, -2A, -2D, and -2G are solutions to the trisection equation in the form of quadratic irrationalities. Since their orders are different, A+B√C, -2A, -2D, and -2G are all different from each other. This means that the trisection equation is a third-degree polynomial equation with at least four distinct solutions, which is impossible, so the trisection equation has no constructible roots.

Since the trisection equation has no constructible roots, and since cos(20°) is a root of the trisection equation, it follows that cos(20°) is not a constructible number, so trisecting a 60° angle by compass and straightedge is impossible.

Conclusion

This article has mostly followed the proof that appeared in The Trisection Problem, an excellent book published in 1971 by the mathematician Robert Yates (during a quick search for information just now, I learned that there is, regrettably, also a serial killer named Robert Yates. Yikes.). This proof is in turn based closely on the original 1837 proof by Wantzel. Although it is a complete proof, one might feel as though there is something missing. One thing that might be particularly tantalizing is the behavior of the constructible numbers: they have the very nice property that you can add them, multiply them, divide them, and subtract them to produce new constructible numbers. Another interesting loose end is their apparent connection to polynomials with rational coefficients.

This is not just a coincidence. Without realizing it, Wantzel used the theory of the abstract algebra of field extension, which would be formalized later in 1846 when the papers of Evariste Galois were posthumously published.

The fact that constructible numbers can be added, subtracted, multiplied, and divded to make new constructible numbers means that the set of constructible numbers combined with the operations of addition and multiplication (along with a few other key properties) forms an algebraic structure called a field. The set of rational numbers ℚ is a subfield of the field of constructible numbers.

Suppose that a, b, and c are rational numbers and that c is not a perfect square, so that √c is irrational. The field extension of ℚ by √c, which is denoted by ℚ(√c), is the set of all numbers of the form p+q√c where p and q are rational, combined with the operations of addition and multiplication. The degree of the field extension ℚ(√c) over ℚ is the degree of the lowest-degree polynomial with coefficients in ℚ that has √c as a root, which is x²-c, so the degree of ℚ(√c) over ℚ is 2. This polynomial is called the minimal polynomial of √c.

It turns out that, in general, the minimal polynomial of an nth degree quadratic irrationality is 2ⁿ, so any constructible number is a root of a polynomial with degree 2ⁿ with rational coefficients. Furthermore, it turns out that x³-3x-1 is the minimal polynomial of 2cos(20°), and three is not a power of two, so 2cos(20°) is not constructible, and therefore neither is cos(20°).

As elegant as that all sounds, it requires an enormous amount of preliminary groundwork in order to make it precise. We will begin this work in the sequel to this article.