Have you thought much about the speed of light and its implications? If not, you really should. Therein lie marvels.

1. We see into the past
2. We all agree on the speed of light
3. Simultaneity is in the eye of the beholder
4. Time slows down
5. Lengths shorten

We See Into The Past

Get a flashlight, stand one foot away from a wall, direct the flashlight at the wall and turn it on. The light beam will show up on the wall, seemingly instantly. Actually, it wasn’t instant. It took approximately a nanosecond. A nanosecond is 10⁻⁹ seconds i.e. a billionth of a second. That’s too short of an interval to be perceptible to a human brain and so it feels like the beam from the flashlight hit the wall instantly.

In a billionth of a second (nanosecond) light travels one foot. This is the time it takes for the light from objects around us in a room to reach us so that we see them almost instantaneously.

In a millionth of a second (microsecond) light travels a thousand feet. The light from a street light a few blocks away takes a microsecond or so to reach us, which still seems instantaneous to us.

In a thousandth of a second (millisecond) light travels 186 miles. That is approximately the distance between Seattle and Portland. Now of course our flashlight won’t be detectable at such large distances (due to its low power). But the time it takes for light to travel hundreds of miles becomes noticeable to us in other ways. For example, if you visit a website on your device in Seattle and the website is served from a data center in Atlanta, the data transfer across the thousands of miles adds tens of milliseconds delay to the loading of the website (compared to if the website was served from a data center in Seattle). In case you’re wondering what data transfer has to do with light, digital data is transmitted as electromagnetic waves in optical fibers (light being electromagnetic waves within a certain band of frequencies that are visible to the human eye).

In one second light travels 186,000 miles or 300,000 kilometers. That’s approximately the distance to the moon. So when we look up at the moon in the night sky, we’re actually seeing it the way it was a second ago (not that the moon is changing every second, but still).

It takes about 8 minutes for the light from the sun to reach us. If the sun were to explode right now (don’t worry, that’s not going to happen till another 5 billion years) we will not know about it till 8 minutes after!

The next time you look up at the night sky, wonder at how you’re looking way into the past. It takes tens of thousands of years for the light from visible stars to reach us. Are some of those stars still around? And if we go beyond the naked eye and consider stars we can detect through telescopes and other instruments, well then we’re looking millions and billions of years into the past!

We All Agree On The Speed Of Light

The speed of light is, stated in various units, 1 foot per nanosecond or 186 miles per millisecond or 300,000 kilometers per second. This is technically the speed in a vacuum (e.g. in space). Light travels a bit more slowly through media such as glass or water, but that’s not relevant for this discussion.

A more relevant issue is according to whose perspective is the speed of light 300,000 kilometers per second? If I’m on a moving bus and there’s a ball on the seat next to me, the ball is stationary (has 0 speed) from my point of view. But as you stand on the street and see the bus drive by, you observe the ball as moving at the same speed as the bus. The speed of the ball is different depending on the observer. What about the headlight beam emanating from the bus? Does it traverse a foot every nanosecond from your perspective or mine? The answer, counterintuitively, is that the headlight beam has the same speed from your perspective as well as my perspective! That is very different from the case of a ball on a bus.

The speed of light is the same from every observer’s perspective, even when the observers are moving rapidly relative to each other. For completeness, I’ll mention the salient detail that each observer must be in an inertial frame (i.e. non-accelerating) but let that detail not obfuscate the main point here. The constancy of the speed of light from every observer’s perspective is a fundamental law of Physics (proposed by Einstein in the year 1905 in a theory known as Special Relativity). And boy does it lead to some mind-blowing effects that we shall explore next.

In the Eye of the Beholder: Simultaneity

I (on a moving bus) see two things happening at the same time. You (standing on the road) should also see those two things happening simultaneously, right? Wrong! This is a result of the speed of light being constant for all observers.

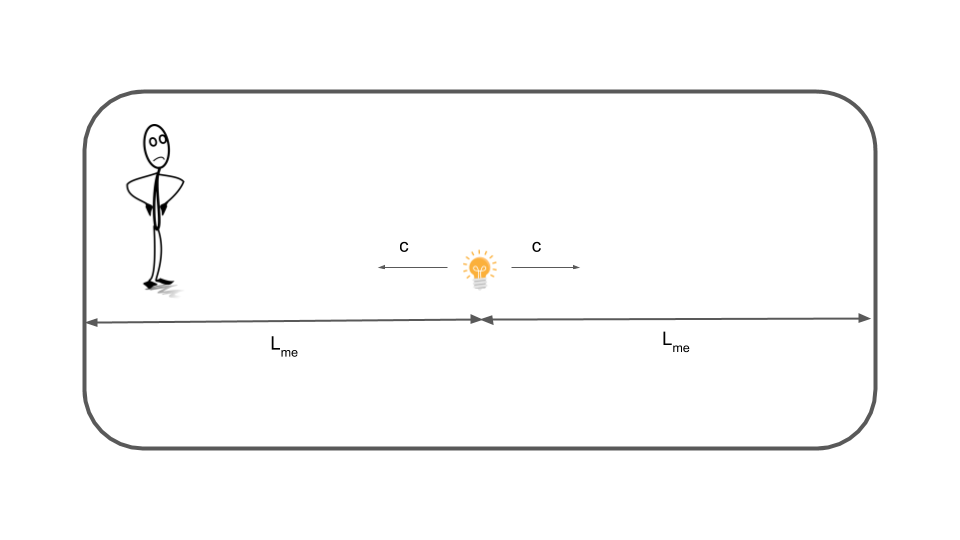
How can we understand this loss of simultaneity across different observers? Well, with some shenanigans on the bus. What happens when inside the bus the driver switches on a light that is exactly half-way between the front of the bus and the rear? Obviously, the light will travel at the same speed (conventionally denoted by ‘c’) towards the front and the back and reach the two ends at the same time (in a few nanoseconds after the light was switched on). If ‘Lₘₑ’ is the distance from the bulb to each end of the bus, then the light will reach each end after a time Lₘₑ / c.

But what do you see while standing on the road as the bus drives by? For you, the interior bus light rays are moving to the front and back of the bus at the same constant speed of light (due to the Physics postulate). But the difference for you is that the back of the bus is moving towards the light at the speed of the bus (let’s call the bus’s speed ‘v’) and the front of the bus is moving away from the light at the speed of the bus. So you see the light reach the back before it reaches the front. If ‘Lₒᵤ’ is the distance you see from the bulb to each end of the bus (which you’d expect to be the same as Lₘₑ, but who knows in all this weirdness), the light reaches the rear end at time Lₒᵤ / (c + v) and then the front end at time Lₒᵤ / (c -v). What I see as happening simultaneously, you see as happening at different times!

Time Slows Down

I, on the moving bus, see my watch’s second hand ticking once every second. I pat the window next to me on every tick. You, on the street, see my bus drive by with me on it, tapping the window periodically. You use your watch to measure the time interval between my taps. You measure one second between taps, right? Wrong! The time between two taps as seen by you is greater than one second! The elapsed time depends on the timekeeper. You will see a moving clock as running slower than a stationary clock.

What shenanigans can I do on the bus this time to help you understand this? I notice that there’s a mirror on the bus’s ceiling. I happen to have a flashlight. I point it up towards the ceiling, switch it on and measure the time it takes for the light to reflect off the mirror and come back to me (the light from the mirror comes back to me when I see the light in the mirror). This only takes a few nanoseconds, so practically speaking it’s not feasible for me to measure such a short time interval. But imagine a tall enough bus and you get the idea. If ‘H’ is the height of the bus then I measure a time of tₘₑ = 2H / c.

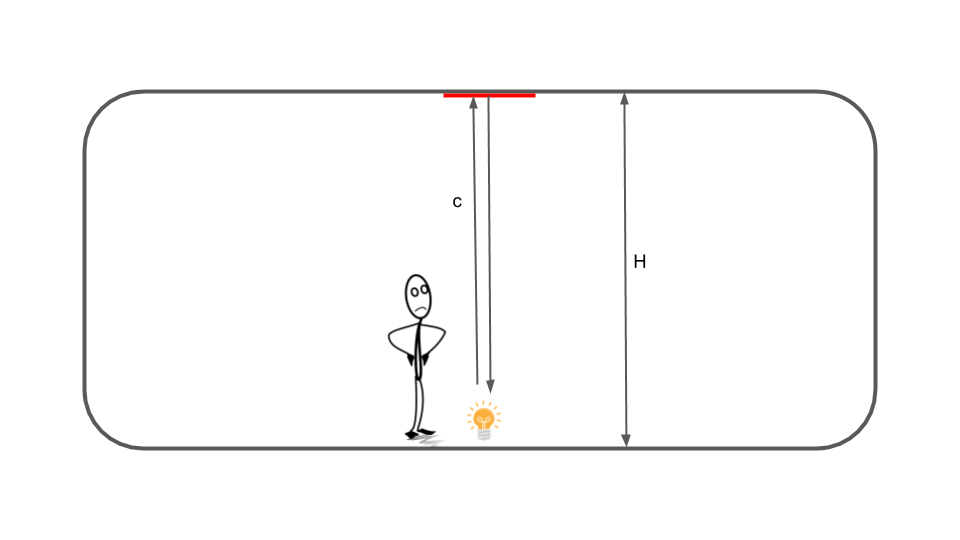
What time interval do you measure for my flashlight beam to travel up to the ceiling, reflect off the mirror and return to me? You will see the light beam go diagonally up to the ceiling with the constant speed of light (again due to the Physics postulate). And then reflect off and return diagonally to me, again with the constant light speed. Since the diagonal paths are longer than the direct vertical paths (remember Pythagoras), you will measure the time interval between me switching on the flashlight and the light beam reflecting back to me to be longer than what I measured. You will see the time interval as dilated compared to what I see.

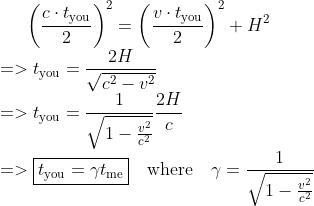
You observe the time passing on my bus as slowed down by a factor of γ, which goes from 1 (when the bus is stationary) to ∞ (when the bus moves at the speed of light). Note that it’s not actually possible for the bus to move at the speed of light because accelerating to that speed would literally require infinite energy. Time dilation and other such effects of Special Relativity become noticeable when γ is noticeably larger than 1. It takes a really high speed for γ to get large. For example, at 87% of the speed of light, γ just about becomes larger than 2. In our everyday lives, we don’t usually encounter such fast objects.

Is this time dilation true or is it just some beguiling trick? It is completely and measurably true. For example, there are unstable, sub-atomic elementary particles called muons that decay to half their quantity every 2 microseconds. These particles are present in cosmic rays bombarding the earth all the time. However, with a 2 microsecond decay period, we would expect hardly any muons to survive the long journey through the earth’s atmosphere before reaching us on the earth’s surface. But in fact, many more than naively expected do survive long enough. The reason for that is time dilation. From our perspective on earth, the muons are moving very fast, so their decay time dilates, leading to significantly longer survival times.

One final note. There are many so-called paradoxes associated with time dilation, casting doubt on its validity (e.g. the twin paradox). Let me reiterate as strongly as possible: time dilation is an observable and measurably true effect and does not actually lead to any paradoxes whatsoever. Each alleged paradox is actually a result of an incomplete or incorrect understanding of Special Relativity.

Lengths Shorten

I measure the length of the moving bus I’m on. From the street, you measure the length of my bus as it passes you by. We both agree on the bus’s length, right? Wrong! Lengths depend on the measurer. You will observe the bus as shorter than what I observe. The length will be shortened by the factor γ we introduced in the time dilation section above.

This time my setup on the bus will use the same light and mirror as that used to illustrate time dilation, except the mirror will be placed at the front of the bus and the light at the back of the bus. If I measure the length of the bus to be ‘Lₘₑ’, then the time it takes for the light to reach the front of the bus and return to the back of the bus will be tₘₑ = 2 Lₘₑ / c.

What do you see standing on the street? Suppose the bus length for you is ‘Lₒᵤ’. Then, the light will take a time Lₒᵤ / (c -v) to reach the front and then Lₒᵤ / (c +v) to reach the back. The forward journey of the light takes longer because the mirror is receding with the motion of the bus and yet the light speeds towards it at the same constant speed. In the reflected journey to the back, the rear of the bus is approaching with the speed of the bus.

You measure the length of the bus as shorter by a factor γ. The closer the bus gets to the speed of light, the shorter the bus gets. Again this is no trick. Length contraction is completely real. It’s just that we’re not used to observing such ultrafast phenomena in our everyday lives.

Some Caveats

1. Don’t try these experiments at home. They are thought experiments. The actual effects of Special Relativity only kick in in a noticeable way when things move unimaginably fast (close to the speed of light). We can’t move a bus that fast. It’s not merely a matter of lack of technology. Even if the technology existed, it would require an exorbitant amount of energy to accelerate a bus anywhere close to the speed of light. Even the fastest spacecrafts travel at a small fraction of the speed of light. Does that mean that these effects are purely theoretical? No. We can clearly and precisely observe the effects of Special Relativity in a variety of scenarios (decay of particles in cosmic rays, smashing of sub-atomic particles in a high energy collider, frequency shifts in the light received from moving stars, etc.).
2. We have been cavalier about ignoring the time it takes for you and me to actually see the events in question (e.g. a light beam striking the front of the bus). To see such an event we technically have to account for the light from it to reach our eyes. But that does not throw a wrench into the works at all. Just imagine that there’s a passenger on the bus exactly where the event occurs and they are the actual observer for that event on the bus. In the case of observations from the street, imagine that there are a bunch of people lined up on the street as the bus drives by so that one of them is exactly lined up with where on the bus the event occurs. The subtle point is that the effects are for each frame of reference (i.e. the bus or the street) and not for a particular person in the frame of reference.
3. The behavior of light is very different from the behavior of everyday objects. Referring back to the previous scenario of me on a bus with a ball lying next to me, the ball is stationary for me while it appears to you (on the street) to be moving at the same speed as the bus. Thus the speed of the ball depends on the observer, unlike how the speed of light is constant for all observers.

Summary

1. The speed of light in a vacuum is the same constant in all inertial (i.e. non-accelerating) frames of reference.
2. Light travels approximately 1 foot per nanosecond or 186 miles per millisecond or 300,000 kilometers per second.
3. Light from distant objects such as stars can take millions of years to make the journey to our eyes so that we see them as they were in the distant past rather than how they are right now.
4. Two events that occur simultaneously for one observer will occur at different times for a relatively moving observer.
5. A moving clock (in fact, all time intervals in the moving frame) will run slower for an observer at rest relative to the moving clock.
6. A moving bus (in fact all lengths in the direction of motion of the moving frame) will measure shorter for an observer at rest relative to the moving bus.
7. The factor by which time dilates and length contracts is denoted by γ. It depends on the speed of the moving frame. When the moving frame is stationary, γ is 1 and there is no time dilation or length contraction. As the speed of the moving frame increases, γ increases from 1. It approaches ∞ as the speed approaches the speed of light.
8. Nothing can move faster than the speed of light. In fact, an object with a mass cannot even reach the speed of light because that would require infinite energy.

If you’re interested in learning more about Einstein’s theory of Special Relativity, a very insightful and clear presentation is in David Morin’s book.