The Simplest Cubic Root

The architectural symmetry of Cubic polynomials supports many root approximation methods, helping intuitive understanding and with a little work, can be transposed to higher orders to minimise more complex math. This post expands on an earlier post, Cubic Polynomials — Using Similar Triangles to Approximate Roots, which promoted the use of Similar Triangles derived from Turning and Inflection Points.

Both methods are very simple and it is difficult to quantify pros and cons in a short post. The latter, being very easily formularised and not requiring calculus beyond finding the Inflection Point Ip is the quicker and simpler of the 2, is best suited when Ip(x)=B/3A<1 and with high x coefficients leading to relatively steeper architecture between turning points.

This post assumes math at high school level.

The Simplest Cubic Root

Strikes a shrinking cord EJ within the range of the Turning Points to intercept the x axis close to the target Root B as shown in Diag 1 with example function y=x³-1.5x²-5x+6. Point G is 180 deg rotationally symmetrical with Root B.

As the chord shrinks it rotates clockwise about the Inflection Point Ip until Intercept E is concentric with point G (undefined) as shown in Diag 2 where Intercept J and Approx Root B both become concentric with Root B. Hence:

Approx Root B=Root B exactly when E=G.

Approximate Root Formula

Because we don’t know the x coordinate of point G we can use a given proximal value, Constant D=6 and use Similar Triangles to calculate Approx Root B Referring to Diag 3 as follows:

Approx Root B=Ip(x)*D/(D-Ip(y) hence;

Approx Root B=0.5*6/(6–3.25)=1.09 which compares with 1.1 actual.

Remaining Roots

To complete the task, the 2nd and 3rd roots are simply ‘downloaded’ from the ‘Extended Quadratic Equation’ I presented in a post article, Cubic Polynomials-A Simpler Approach.

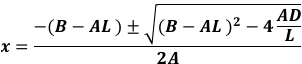
Where Factor L=-1.09, which we have just found, A and B are usual cubic coefficients and D the constant.

Summary

This post introduces Similar Triangles within the symmetrical architecture of the Cubic polynomial to find the ‘Simplest Root’. The method strikes a cord through the Inflection Point Ip, requiring only basic math for a sound root approximation method.

More importantly I have tried to nurture intuitive learning, relating the math with the graph; making math work for you; not you for it!