The Poincaré Conjecture (1904)

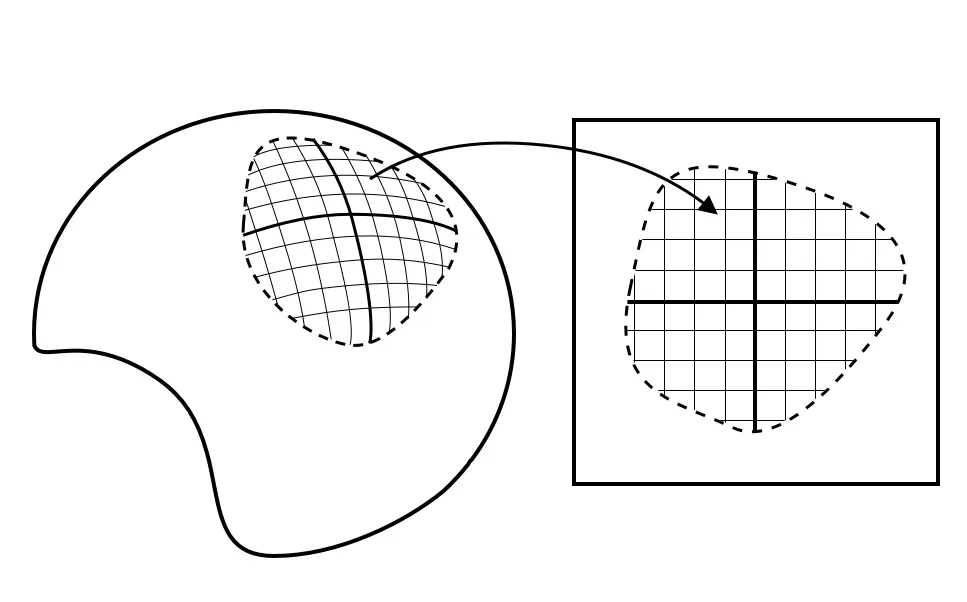
What is the shape of the universe?

Let’s start off easy. We know the shape of the earth. It is more or less spherical. Next, our galaxy, the Milky Way. Somewhat surprisingly to Edwin Hubble (1889-1953) who discovered it, it is barred spiral shaped, i.e. the shape of a disk with spiral arms. What about the observable universe? Is it spherical? It certainly seems to be, as it is expanding outwards and light is more redshifted the further we look. What about the global universe, beyond the horizon of what is observable to us?

The answer is, we do not know. We can conjecture. It may be finite or infinite, with or without boundary, with or without curvature, simply connected like a sphere, or multiply connected like a donut. What we do know, is that it appears to be expanding. To what? We do not know. But we can conjecture. 

Introduction

The shape of the universe as it was, is now and may become in the future is very hard for us to discern empirically. Einstein helped us somewhat by showing us that matter and energy (three-dimensional phenomena) in fact may interact with the a four-dimensional phenomenon: time. In this interaction, spacetime may be warped by the presence of mass/energy. Taken together, as far as we can tell, we live in a four-dimensional universe that is susceptible to deformation such as stretching, twisting and bending. That’s where Henri Poincaré and the invention of topology comes in.

Let’s get back to basics. We all know informally that a circle on a plane is the one-dimensional circumference of a two-dimensional disk. Up one dimension, we also know intuitively that the two-dimensional surface of a three-dimensional ball is called a sphere. Up one more dimension however, and our intuition already fail us completely. What is the three-dimensional equivalent of an object embedded in four-dimensional space? Well, equally as real as the former two, the three-dimensional boundary of a four-dimensional ball in four-dimensional Euclidean space is in mathematics known as a glome, or 3-sphere. I would visualize it for you here if I could, but I can’t.

In mathematics the three objects (circle, sphere and glome) are closely related and known as 1–, 2- and 3-spheres. Such n-spheres are generalizations of the ordinary sphere to spaces of arbitrary dimension. In topology, n-spheres are treated as n-dimensional manifolds, which are topological spaces that locally resemble Euclidean (flat) space near each point. More precisely:

Definition of a manifold
Each point of an n-dimensional manifold has a neighborhood that is homeomorphic to the Euclidean space of dimension n.

In thinking about what a manifold is, author Sylvia Nasar in her book A Beautiful Mind provides a useful perspective:

Think of yourself shrunk to the size of a pinpoint, sitting on the surface of a doughnut. Look around you, and it seems that you’re sitting on a flat disk. Go down one dimension and sit on a curve, and the stretch nearby looks like a straight line. Should you be perched on a three-dimensional manifold, however esoteric, your immediate neighborhood would look like the interior of a ball. In other words, how the object appears from afar may be quite different from the way it appears to your nearsighted eye.

Before topology (1752–1895)

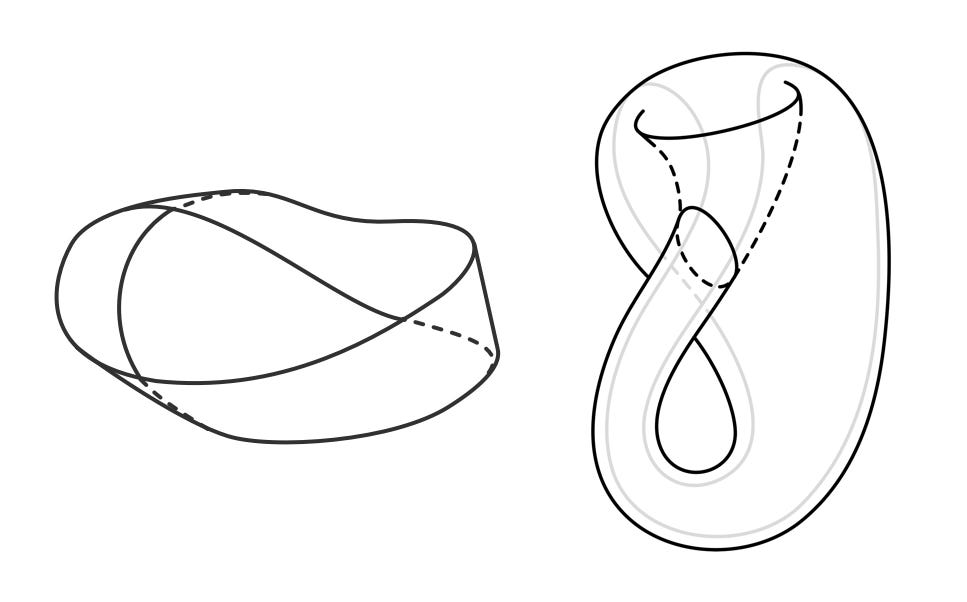
John Stillwell in his wonderful book “Papers on Topology: Analysis Situs and Its Five Supplements” (2010) claims, not unsubstantiated, that only a single (universal) topological concept had been defined before Henri Poincaré (1854-1912). This concept was the now well known Euler characteristic (χ) given by Euler’s polyhedron formula V — E + F = χ, where V stands for vertices, E for edges and F for faces. The Euler characteristic both for the sphere and convex polyhedra such as e.g. the Platonic solids is two. In his investigations into the topological classification of such surfaces, Möbius in 1863 showed that all closed surfaces in R³ — that is, orientable surfaces , are classified by their Euler characteristic.

Stillwell (2010) also mentions the relevant contributions of figures such as Gauss (1827) and Bonnet (1848) and their discovery of the average curvature of smooth surfaces, as well as the study of algebraic curves by Riemann (1851). In addition, he mentions the relevancy of the study of “pits, peaks and passes” on surfaces in R³ by Cayley (1859), adding however that it was not until Enrico Betti (1871) that real strides were made towards studying such concepts in arbitrary dimensions.

Betti defined what would later be known as the Betti numbers P₀, P₁, P₂, … , in all dimensions. In algebraic topology, informally, the kth Betti number refers to the number of k-dimensional holes on a topological surface, or stated differently, “the maximum number of cuts that can be made without dividing a surface into two separate pieces” (Gardner, 1984 p. 9–10). For 0-dimensional, 1-dimensional and 2-dimensional sets of simplical complexes (sets composed of points, line segments, triangles and their n-dimensional counterparts), the Betti numbers have the following definitions:

• P₀ is the number of connected components
• P₁ is the number of one-dimensional or “circular” holes
• P₂ is the number of two-dimensional “voids” or “cavities”

A torus for instance has Betti numbers P₀ = 1, P₁ = 1, P₂ = 1 because it is a single connected surface component with two circular holes that connect and form a surface that encloses a single cavity. The second Betti number P₁ is also called the genus of a surface, informally “the number of holes it has” and is equal to the Euler characteristic χ. As John Milnor (1931-) wrote in the official statement of the Poincaré Conjecture for the Clay Mathematics Institute’s Millenium Prize:

The topology of 2-dimensional manifolds, or surfaces was well understood in the 19th century. [...] Any such surface has a well-defined genus g ≥ 0, which can be described intuitively as the number of holes.

Milnor presents this informal introduction to manifolds and topology with a simple sketch of three figures of genus 0, 1 and 2 (see below).

Before Poincaré, as both Milnor and Stillwell argue, the only topological concept that was well defined was indeed the theory of closed surfaces, that is, so-called 2-manifolds. Their properties are that they are compact and without boundary. The classification theorem of closed surfaces states that any connected closed surface is homeomorphic to some member of one of the three families: 1. The sphere; 2. The connected sum of g tori for g ≥ 1; and 3. The connected sum of k real projective planes for k ≥ 1. Milnor ends his discussion of (such much simpler) surfaces with the statement: “The corresponding question in higher dimensions is much more difficult.”

Henri Poincaré would be the first person to try to conduct a similar study as had been done for 1- and 2-manifolds, with three-dimensional manifolds — namely to investigate whether such objects could be shown to be homeomorphic with one other.

Henri Poincaré (1854–1912)

Henri Poincaré was born on the 29th of April 1854 in the Cité Ducale neighbourhood of Nancy, France to Leon Poincaré and Eugénie Launois. His father was a professor of medicine at Nancy-Université and his mother a homemaker who tended to Henri and homeschooled him when he fell ill from diphtheria during his childhood.

In 1862 at the the age of eight he entered into the Lycée, where he spent nine years, excelling in every topic he studied. The first recognitions of his talent came from a mathematics teacher who described him as a “monster of mathematics” and from first prizes in the concours général, a competition between the top students from all Lycée schools across France. In addition to mathematics, he also excelled in writing composition. His poorest subjects were, not-uncharacteristically for future mathematicians, music and physical education. His poor eyesight and tendency towards absentmindedness may however have explained these difficulties, according to Carl (1968). In 1871 he graduated from Lycée with a B.Sc. in letters and science and joined his father on the front in the Franco-Prussian War, serving in the Ambulance Corps.

Following the war, in 1873 Poincaré next entered into university at the École Polytechnique where he studied mathematics under Charles Hermite (1822-1902), publishing his first paper at the age of 22 entitled Démonstration nouvelle des propriétés de l’indicatrice d’une surface (“New demonstration of the properties of surface indicators”). In 1875, in addition to his studies in mathematics he entered into a mining engineering program at the École des Mines, graduating in 1879 with the degree of engineer. He immediately put his new degree to use, joining the Corps des Mines as an inspector. Simultaneously, he was preparing for his Doctorate of science in mathematics on the topic of differential equations at Collegé de Sorbonne at Université de Paris. His dissertation was entitled Sur les propriétés des fonctions définies par les équations aux différences partielles (“On the properties of functions defined by partial difference equations”) and was completed in 1879.

Despite graduating doctorat d’État in mathematics, Poincaré continued working as a mining engineer, in charge of the northern railway development from 1881 to 1885. He meanwhile also began teaching mathematics at his alma mater, the Collegé de Sorbonne and continued conducting research, developing a new branch of mathematics called the ‘qualitative theory of differential equations’. Investigations in this branch involves studying the behavior of differential equations by means other than finding solutions. In addition to this and his later work on topology, through the course of his career Poincaré also worked on analytic functions of several complex variables, abelian functions, algebraic geometry, hyperbolic geometry, number theory, the three-body problem, diophantine equations, electromagnetism, relativity theory, philosophy and group theory, earning Poincaré the nickname “The Last Universalist”.

Poincaré’s work on topology

Poincaré began working on what is now considered to be the foundation of topology and algebraic topology in the 1890s with the work Analysis Situs (1895) and five later supplements (1899; 1900, 1902a, 1902b, 1904). The term ‘Analysis Situs’, or “the geometry of position” was inspired from Gottfried Leibniz (1646-1716)’ reference to the term over 150 years prior in his writings from 1672–76 (De Risi, 2018 p. 247).

Poincaré declared his motivation for establishing a new branch of mathematics devoted to topology, or ‘the study of geometric objects undergoing deformation’, “rubber-sheet geometry”, in Analysis Situs with the statement (1895):

“On a bien souvent répété que la Géométrie est l’art de bien raisonner sur des figures mal faites; encoreces figures, pour ne pas nous tromper, doivent-elles satisfaire à certaines conditions; les proportions peuvant être grossièrement altérées, mais les positions relatives des diverses parties ne doivent pas être bouleversées.”

Namely, that there was a need for mathematicians to be able to determine with certainty that our encoreces figures ‘badly drawn figures’ must satisfy certain conditions such that even though their ‘proportions may be grossly altered’, that the ‘relative positions of the different parts must not be upset’. The statement aligns closely with the modern day definition of topology:

Topology is the study of the properties of a geometric object that is preserved under continuous deformations, such as stretching, twisting, crumpling and bending, but not tearing or gluing.

On Analysis Situs (1892)

In his first paper (letter, really) on topology, Poincaré sets out to motivate the first real primer on topology, Analysis Situs (1895). He does so by referring to the Betti numbers introduced about 20 years earlier. His argument, or question to the reader, is whether Betti numbers actually suffice to determine the topological classification of a manifold. To show why they may not, he introduced the concept of a fundamental group π₁. Informally, a fundamental group may be thought of in the following way:

Start with a space (e.g. a surface), and some point in it, and all the loops both starting and ending at this point — paths that start at this point, wander around and eventually return to the starting point. Two loops can be combined together in an obvious way: travel along the first loop, then along the second. Two loops are considered equivalent if one can be deformed into the other without breaking. The set of all such loops with this method of combining and this equivalence between them is the fundamental group for that particular space.

He next describes a family of three-dimensional manifolds and shows that certain of these manifolds have the same Betti numbers, yet belong to different fundamental groups (Stillwell 2010, p. 6). From this, he argues that if the fundamental group is a topological invariant (a property preserved while undergoing homeomorphisms), that Betti numbers alone cannot distinguish three-dimensional manifolds from one another.

Analysis Situs (1895)

The later Poincaré conjecture (1904) in fact did not exist in 1895, as according to Stillwell (2010), Poincaré at this point likely thought it obvious that all simply-connected n-dimensional closed manifolds would be homeomorphic to the n-sphere, i.e. that all such manifolds would preserve their topological properties if deformed to the shape of a sphere in n-dimensions. After all, the same result had been known to be true for 1- and 2-dimensional manifolds since the time of Riemann.

The Analysis Situs sets out, rather, to revise and supplement Betti numbers in search of a more solid foundation, given his own argumentation from three years prior. The paper works towards this goal by several paths. He begins by introducing, as often is the case in research, a justification for why the work is valuable, stating that “The geometry of n dimensions is a real object, nobody doubts that nowadays. Figures in hyperspace are as susceptible to precise definition as those in ordinary space, and even if we cannot represent them, we can still conceive of them and study them. So if the mechanics of more than three dimensions is to be condemned as lacking in object, the same cannot be said of hypergeometry” (Stillwell, 2010).

La Géométrie à n dimensions a un objet réel; personne n’en doute aujourd’hui

Among the multiple great discoveries in Analysis Situs, Poincaré presents the foundations for what would later be called homology theory, a way of associating a sequence of algebraic structures such as abelian groups or modules with other mathematical objects such as topological spaces. He arrives there by setting up a system for computing Betti numbers by assuming that every manifold can be decomposed into cells which are homeomorphic to simplices (essentially tetrahedrons in n-dimensions), reading off linear equations he called homologies and computing the corresponding Betti numbers by linear algebra (Stillwell 2012, p.557). As Scholz (1980) put it: “The first phase of algebraic topology, inaugurated by Poincaré, is characterized by the fact that its algebraic relations and operations always deal with topological objects.”

Using his new homology theory, Poincaré next provides the Poincaré duality theorem for the Betti numbers for an n-dimensional manifold, by considering the dual of the cell decomposition. The duality theorem states that Betti numbers of the same distance from the ‘ends’, i.e. top and bottom dimensions, are equal. In particular, for a 3-manifold the 2-dimensional Betti number equals the 1-dimensional Betti number (Stillwell, 2012).

Later in the same paper, Poincaré also provides a generalization of the Euler polyhedron formula to arbitrary dimensions, and relates it to his homology theory (Stillwell, 2010). He also gives new examples of fundamental groups which establish that π₁ is a stronger invariant than the Betti numbers, by identifying opposite faces of an octahedron with the same Betti numbers as the 3-sphere, but again, a different fundamental group (namely the cyclic group). The take-away from his discoveries is that for 0-, 1- and 2-dimensional manifolds, the Betti numbers suffice to distinguish them between one another, but that for three-dimensional manifolds, the fundamental group becomes important. How important, Poincaré at this point (1895) cannot answer.

In retrospect, as Stillwell (2010) writes, because of Poincaré’s construction of homology theory and the establishment of the fundamental group, Analysis Situs is rightly regarded as the origin of algebraic topology. As for homology theory, the importance of its establishment was that it revealed that there is an algebraic structure giving rise to the Betti numbers (and so the Euler characteristic). The discovery of the fundamental group highlighted the lacking power of Betti numbers as an indicator of manifolds’ properties.

First and second supplements to Analysis Situs (1899–1900)

Analysis Situs, although brilliantly inventive, was provided not without confusion or error (Stillwell, 2010). Exploring in somewhat of a ‘no man’s land’, Poincaré only later came to discover that Betti numbers were only part of the story, an oversight which he would be made aware of three years later with the thesis of a Danish doctoral student, Poul Heegaard (1871-1948).

Poincaré wrote his first supplement, entitled Complément a l’analysis situs (“Supplement to the Analysis Situs”) in 1899. The paper was motivated by the discovery of Heegaard (1898) that Poincaré’s new definition of Betti numbers could be shown to be in conflict with his duality theorem. Heegaard contrasts the example of the real projective space RP³ with the example of the 3-sphere and shows that Poincaré had failed to account for the effects of torsion, “twisting”. After first moving towards a more combinatorial theory of homology, in which manifolds are assumed to have a polyhedral structure, in his supplements (sometimes referred to by Poincaré as complements) Poincaré eventually revises his homology theory to produce torsion numbers in addition to Betti numbers, proved to be invariant using the so-called Hauptvermutung argument (any two triangulations of a triangulable space have subdivisions that are combinatorially equivalent), now known to be false. The inclusion strengthened homology theory to be able to distinguish between manifolds undergoing torsion from one another (including RP³ and the 3-sphere). Poincaré indeed coined the term ‘torsion’ through a discussion of non-orientable surfaces such as the Möbius band (Stillwell, 2010).

In the second supplement paper (1900), Poincaré’s now more robust homology theorem emboldens him to end his paper with the statement:

“Each polyhedron which has all its Betti numbers equal to 1 and all its tables Tq orientable is simply connected, i.e. homeomorphic to a hypersphere”

Effectively conjecturing that

“Any three-manifold with trivial homology is homeomorphic to the 3-sphere”

A first (and incorrect) Poincaré conjecture about the topological properties of three-dimensional bodies undergoing deformation.

Third and fourth supplements to Analysis Situs (1902)

In terms of 3-manifolds and the later Poincaré conjecture, the relevance of the third and fourth supplements (other than expanding on Poincaré’s homology theory and algebraic topology) is in their study of torus bundles, which are shown to arise naturally in the study of algebraic curves, the focus of the third supplement “On certain algebraic surfaces” (1902a) (Stillwell, 2010).

Fifth supplement to Analysis Situs (1904)

Poincaré published his fifth and final supplement to his 1895 Analysis situs paper in 1904. The paper regards three-dimensional manifolds (such as the glome from our introduction) and is entitled Cinquième complément à l’analysis situs. The paper is now known for Poincaré’s study of whether 3-dimensional manifolds can be described by the same distinguishing feature as 2-dimensional manifolds, namely that every simple closed curve in the sphere can be deformed continuously to a point without leaving the sphere. As Poincaré himself writes in his introduction:

“This time I confine myself to the study of certain three-dimensional manifolds, but the methods used without doubt are of more general applicability. I shall devote considerable space to certain properties of closed curves which can be traced on closed surfaces in ordinary space.”

The paper sets out, not with an investigation of simply connected, closed 3-manifolds, but rather, with a study of the differences between homology and the more extensive homotopy theory, in the case of curves on surfaces via the study of fundamental groups (Stillwell, 2012). The result is an interesting geometric algorithm to decide whether a curve on a surface is homotopic to a simple curve, the first known case of applying geometrization to topology, which would later would inspire the work of Dehn (1910; 1912a; 1912b; 1922), Nielsen (1927; 1929; 1932), and Thurston (1979). The paper goes on to investigate various other properties of curves on surfaces and their role in constructing three-dimensional manifolds (Stillwell, 2010).

In the final pages of the paper, Poincaré’s investigating leads to a new discovery, the homology sphere called the ‘Poincaré homology sphere’, a 3-manifold with the same homology as the 3-sphere, but a different fundamental group. An existence proof, the discovery immediately rejects Poincaré’s initial conjecture about the relationship between 3-manifolds and 3-spheres from 1902. He constructs the object by joining two “filled” bodies of genus 2 (so-called handlebodies) so that certain carefully chosen curves on one of them become identified with curves with very particular properties on the other. From this, he obtains a three-dimensional manifold whose fundamental group can be written down simply in terms of its generators and relations. “By some miracle” (Stillwell, 2012), the fundamental group of the Poincaré homology sphere turns out to have generators a and b whose relationship is:

a⁴ba⁻¹b = b⁻²a⁻¹ba⁻¹ = 1

A seemingly utterly asymmetric object, the homology sphere is in fact exceptionally symmetric and easily shown to be nontrivial (of dimension 2 or higher) by setting (a⁻¹b)² = 1, which maps it to the 60-element icosahedral group a⁵ = b³ = (a⁻¹b)² = 1. Simultaneously, by allowing the generators to commute, one finds that the object collapses to a single element, 1, showing that the homology of the manifold must be trivial. Hence, the homology sphere is in fact a closed three-dimensional manifold with trivial homology but a non-trivial fundamental group. The discovery of this certainty re-demonstrated for Poincaré the power of his fundamental group over homology for distinguishing 3-manifolds, and put a final nail in the coffin for his first and flawed conjecture about the usefulness of homology in demonstrating that all 3-manifolds are homeomorphic to the 3-sphere.

Poincaré ends the fifth supplement to his Analysis Situs with a question:

“Is it possible for the fundamental group of a manifold to reduce to the identity without the manifold being simply connected?”

..and an obfuscation:

“This question would carry us too far away.”

The Poincaré conjecture (1904)

Every simply connected, closed 3-manifold is homeomorphic to the 3-sphere.

This is the conjecture made by Poincaré at the end of his 1904 supplement to Analysis Situs, regarding the characterization of three-dimensional manifolds as homeomorphic to the 3-sphere. In modern terms, precisely, the Poincaré conjecture states (Milnor, 2004):

If a smooth compact 3-dimensional manifold M³ has the property that every simple closed curve within the manifold can be deformed continuously to a point, does it follow that M³ is homeomorphic to the sphere S³?

Informally, the conjecture asserts that if every simple closed curve within the manifold, such as a loop, can be deformed (tightened) to a single point, then it must be a 3-sphere. Because we unfortunately cannot efficiently visualize 3-dimensional manifolds, analogous 2-manifolds are shown in the figure below with loops in blue and green. As we can see any loop (connected path) on the sphere can be tightened and pulled off the surface by sliding them up or to the side. On the torus however, although the blue loop can be tightened and slid off, the green loop cannot, without slicing the torus. Hence, the torus is not homeomorphic to the sphere.

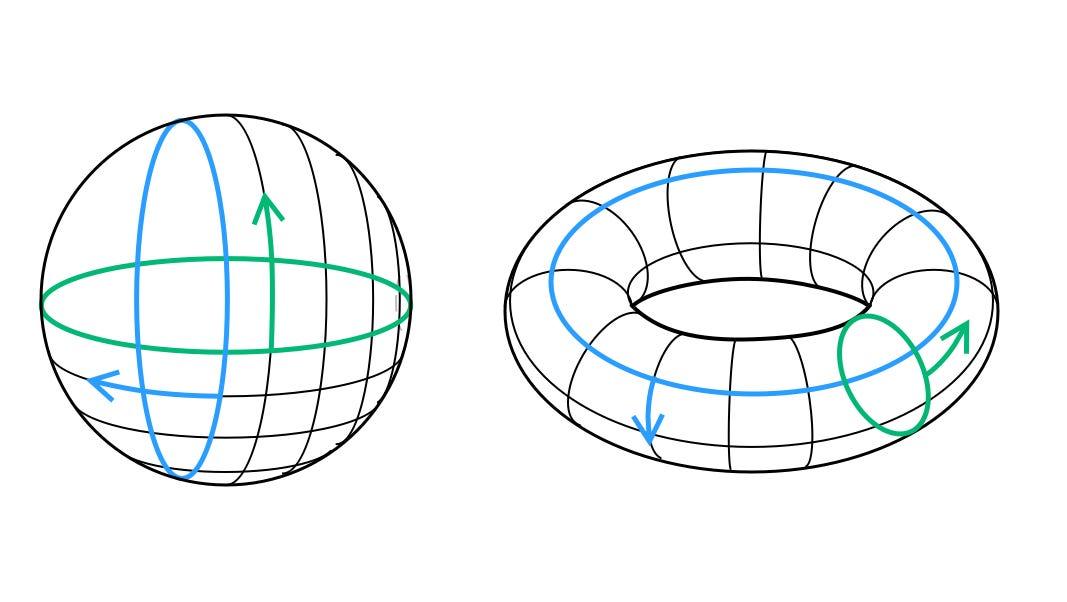
If you tie a slipknot around a soccer ball, you can easily pull the slipknot closed by sliding it along the surface of the ball. But if you tie a slipknot around a bagel through the hole in its middle you cannot pull the slipknot closed without tearing the bagel.

- Excerpt, Manifold Destiny* by Masha Gessen

Implications of the Poincaré Conjecture

As you’ve probably figured out by now, the connection between Poincaré’s conjecture and the shape of the universe is implicit, yet still very salient. Stated simply, if the universe is a simply connected, closed 3-manifold, it is homeomorphic to the sphere. That means, that although the universe may indeed be in the shape of a 3-torus, if so, we know it can never expand to the shape of a 3-sphere or visa versa.

The above narration of the history of Poincaré’s conjecture is based in large part on the wonderful book Papers on Topology: Analysis Situs and Its Five Supplements* by John Stillwell (2010), simplified here and there for easier reading. For the casual reader interested in learning more about the Poincaré Conjecture, I especially recommend Stillwell’s paper Poincaré and the Early History of 3-manifolds (2012). The most comprehensive biography about Henri Poincaré is Jeremy Gray’s Henric Poincaré - A Scientific Biography*.

References

• Carl, B. 1968. A History of Mathematics: Henri Poincaré, John Wiley & Sons.
• De Risi, V. 2018. The Oxford Handbook of Leibniz.
• Milnor, J. 2004. The Poincaré Conjecture. Clay Mathematics Institute
• O’Shea, D. 2007. The Poincaré Conjecture
• Poincaré, H. 1895. Analysis Situs
• Poincaré, H. 1899. Supplement to Analysis Situs. Rendiconti del Circulo Matematico di Palermo 13, pp. 285–343.
• Poincaré, H. 1900. Second supplement to Analysis Situs. Proceedings of the London Mathematical Society 32, pp. 277–308.
• Poincaré, H. 1902a. On certain algebraic surfaces; Third supplement to Analysis Situs. Bulletin de la Société Mathématique de France 30. pp. 49–70.
• Poincaré, H. 1902b. Cycles on algebraic surfaces. Fourth supplement to Analysis Situs. Journal de Mathématiques 8. pp. 169–214.
• Poincaré, H. 1904. Fifth supplement to Analysis Situs. Rendiconti del Circulo Matematico di Palermo 18. pp. 45–110.
• Stillwell, J. 2010. Papers on Topology: Analysis Situs and Its Five Supplements. (Vol. 37). American Mathematical Society.
• Stillwell, J. 2012. Poincaré and the Early History of 3-manifolds

* This essay contains Amazon Affiliate Links