The Discovery Of The First Irrational Number
How Hippasus of Metapontum ended up being drowned at sea for coming up with a groundbreaking proof.

Ancient Greek philosopher Pythagoras and his followers were the first practitioners of modern mathematics. They understood that mathematical facts weren’t laws of nature but could be derived from existing knowledge by means of logical reasoning. But even good old Pythagoras lost it when Hippasus, one of his faithful followers, showed him an incredible proof. His student had just found the first irrational number! Let’s see how it all happened.
How a Monochord Misled the Pythagoreans
A monochord is an ancient musical instrument. It’s somewhat similar to a guitar, but it only has one string. The Pythagoreans used it for a variety of scientific experiments.

Their monochord had a moveable bridge which allowed them to set up and play all kinds of different musical intervals. At some point, the Pythagoreans realized that intervals with simpler ratios sounded significantly nicer than those with more complicated ones. For example, an interval of 1/2 was way more pleasing to the ear then, let’s say, 67/193.
This rather surprising finding let the Pythagoreans to believe that absolutely everything can be quantified using a ratio of integers. In other words, they believed that every point on the number line corresponds to a rational number. It simply made a lot of sense to them until Hippasus visited Pythagoras and dropped a bombshell.
Hippasus’ Proof
The sign of the Pythagoreans was a star with five jags. Connecting all adjacent jags creates a regular pentagon. One day while playing around with such pentagons, Hippasus constructed a smaller pentagon from a bigger one.

The smaller blue pentagon is mathematically similar to the larger red one. They are the exact same objects drawn at different scales. He then came up with the following simple equation.

The equation is obviously correct, both sides express the same ratio of diagonal length to side length. The left side does it for the larger red pentagon, the right side for the smaller blue one. Assuming d and s to be natural numbers instead of integers also makes sense because we’re talking about lengths. Hippasus now came up with the following line of argumentation.
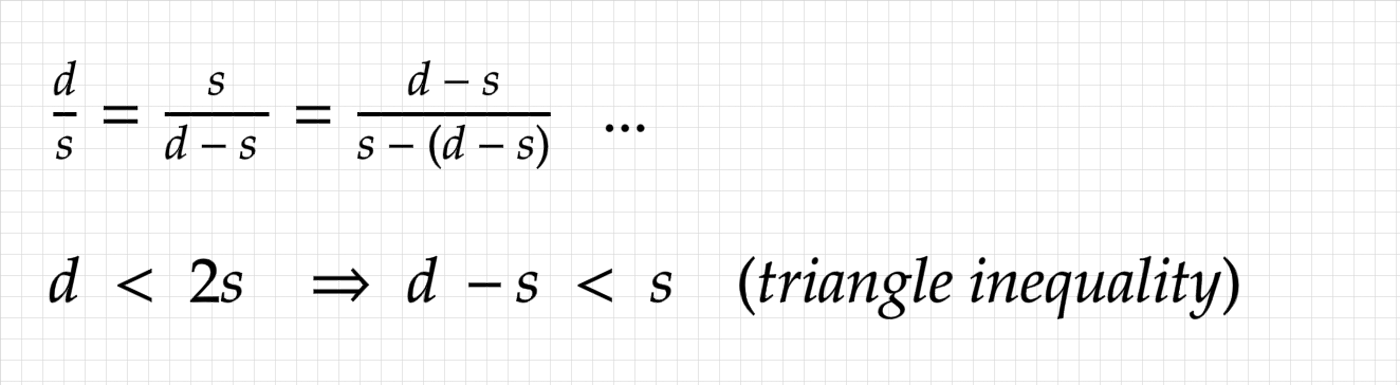
First of all, he assumed that the ratio of diagonal length to side length is a rational number. He then argued that smaller and smaller pentagons can be constructed. The smaller pentagon from the last construction simply serves as the larger one for the next construction. All pentagons’ ratios of diagonal length to side length are the same, and all of these ratios can be expressed using only d and s. Because of triangle inequality, every newly constructed pentagon’s ratio would have a smaller denominator than the previous one.
This however will become impossible at some point because the set of natural numbers has a smallest element which happens to be zero. Therefore, the initial assumption must be wrong and the ratio of diagonal length to side length can’t be a rational number. Today, this method of proof is known as infinite descent.
Dead Men Tell no Tales
Once he had double-checked his proof, Hippasus showed it to Pythagoras. Even though Pythagoras certainly didn’t have any problems understanding the proof, he was extremely outraged that his world view had just been shattered. The story goes that he had poor Hippasus drowned at sea. While it’s not clear if this actually happened or not, there can’t be much debate that the discovery of the first irrational number was a groundbreaking achievement.





