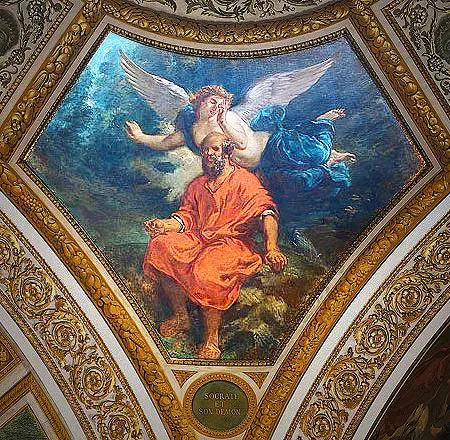
There is a rich history of mathematicians, philosophers, and other thinkers invoking demons (real or imaginary) in their reasonings. The tradition goes back at least to the iconic ancient Greek philosopher Socrates, who we know through the works of Plato, Xenophon, and Aristophanes. In Plato’s dialogues, Socrates claims to have a personal δαίμων or daemon who would speak to him and let him know when he was wrong. In Greek mythology a δαίμων was a kind of minor deity or spirit, not necessarily good or evil. It was only when the Greek word was used in the New Testament that the demon became known as the force of evil which it is now recognized. To Socrates, his daemon served more as a guardian angel or spiritual conscience than as malevolent oppressor.

This idea of a personal demon is discussed by mathematician Jacques Hadamardin his seminal work on the psychology of invention in the mathematical field. In this work he discusses a recurring phenomena, where great mathematicians (Henri Poincaré is a main example) discover revolutionary results suddenly, as if by revelation from their unconscious mind. He speaks of a part of the mind which keeps secrets from us:

In such cases, we must admit that some parts of the mental process develop so deeply in the unconscious that some parts of it, even important ones, remain hidden from our conscious self. We come very near the phenomena of dual personality such as were observed by psychologists of the nineteenth century. Even intermediaries seem to have existed between the two kinds of phenomena. I think of Socrates’ ideas being suggested to him by a familiar demon or also of the nymph Egeria whom Numa Pompilius used to consult frequently.

Hadamard goes on to compare these cases to the case of Gerolamo Cardano (1501–1576), who was the first to calculate using imaginary numbers, and reported receiving ideas from a strange voice.

Moving on to the scientific revolution and beyond, philosophical and mathematical demons continued to appear on the scientific landscape. There are many legendary figures who have personal demons associated with their names. These demons have helped us learn the limits of what we can know, provided substance to thought experiments about the universe and probability, and served as a challenge to the laws of nature themselves. In what follows, we will become acquainted with these famous demons, and in doing so become acquainted also with a sizeable part of the history of western physics. As a conclusion, we will pose a mystery related to an unknown demon of a mathematical giant.

Descartes’ Demon

On November 10th, 1619, the 23 year old René Descartes had a series of visions and dreams that could be said to have altered the course of human thought forever. In Descartes’ fever dreams, he gained a profound new idea:

The vision was preceded by a state of intense concentration and agitation. Descartes’ overheated mind caught fire and provided answers to tremendous problems that had been taxing him for weeks. He was possessed by a Genius, and the answers were revealed in a dazzling, unendurable light. Later, in a state of exhaustion, he went to bed and dreamed three dreams that had been predicted by this Genius…

What was the idea that Descartes saw in a burning flash? He tells us that his third dream pointed to no less than the unification and illumination of the whole of science, even the whole of knowledge, by one and the same method: the method of reason.

Excerpt from Descartes’ Dream, Philip J. Davis & Reuben Hersh

Descartes would soon go on to invent analytic geometry, the method of associated geometric figures with algebraic equations in a coordinate plane that we will forever call Cartesian. The analytic geometry is the perfect tool to fulfill Descartes’ dream. The word geometry comes from the Greek γεωμετρία with literally means “measurement of the earth”; it had been traditionally practiced in the way of Euclid, where figures were drawn using compass and straight-edge and theorems demonstrated by construction through drawing. Descartes’ new method allowed these geometrical figures to be translated into sequences of symbols, algebraic equations which could then be manipulated and solved using pure logic. In this way, real world problems relating to matter in space could be converted into problems of pure reason. While this may seem trivial now to those of us who have been dealing with Cartesian coordinates since early grade school, the idea was truly revolutionary.

Descartes’ new mathematical tool fit perfectly in to his general philosophy of rationalism, the idea that all knowledge could be deduced from pure reason. Descartes would also formulate critical ideas which would lead future historians to dub him the father of modern philosophy. It is in this context in which he invokes his evil demon.

Descartes’ demon first rears its head in his 1641 work Meditations on First Philosophy. This work contains a series of meditations designed to lead the reader to a firm foundation of philosophical belief. In the first of these, “What can be called in to doubt”, Descartes challenges the foundations of all beliefs or “knowledge” that the reader holds, asking us to determine if we really know what we think we know. It is the role of the demon to cast this doubt:

I will suppose not a supremely good God, the source of truth, but an evil genius, supremely powerful and clever, who has directed his entire efforts to deceiving me. I will regard the heavens, the air, the earth, colors, shapes, sounds, and all external things as nothing but the bedeviling hoaxes of my dreams, with which he lays snares for my credulity. I will regard myself as not having hands, or eyes, or flesh, or blood, or any senses, but as nevertheless falsely believing that I possess these things. I will remain resolute and steadfast in this meditation, and even if it is not within my power to know anything true, it is certainly within my power to take care resolutely to withhold my assent to what is false, lest this deceiver however powerful, however clever he may be, have any effect on me.

In this way Descartes’ demon strips him of all knowledge and beliefs, leaving him in an extreme state of skepticism.

It is from this place of extreme skepticism that Descartes arrives at perhaps his most famous dictum:

Cogito, ergo sum.
Je pense, donc je suis.
I think, therefore I am.

It is with this that Descartes overcomes his demon. While the powerful evil entity can call in to question the reality of all sense experience, all intuition, even logical and mathematical truths, there is one thing that Descartes is sure of; he exists, because he is thinking, and possibly being deceived. One must exist in order to be deceived by an evil demon!

It is upon this unshakeable rock that Descartes is able to build his temple, the rest of his metaphysics. From thereon, he deduces that an omnipotent and omnibenevolent God must exist, and comes to the conclusion that humans (and the rest of the world) are made of two distinct types of stuff: mind and body, or spirit and matter. These ideas go on to have their own fascinating history, but that is all the time we have to dwell on Cartesian philosophy. We have other demons to discover.

Laplace’s Demon

Descartes’ mathematical rationalism spurred on an explosive scientific revolution which led to Isaac Newton tempering this rationalism with empiricism, inventing calculus, and unifying the physics of heaven and earth under one mechanistic mathematical philosophy. With the new tools of algebraic geometry and calculus, the next generation of mathematical physicists ran wild, applying these skeleton keys to the solution of myriads of problems. Perhaps the prince of these next generation thinkers was Pierre-Simon, marquis de Laplace(1749–1827). Being an exceptionally powerful mathematician, he is sometimes called the Newton of France, since he applied calculus to a huge range of problems involving astronomy, gravitation, and geodynamics.

Unlike Descartes, who solved his philosophical problems with recourse to an omnibenevolent God, Laplace was probably an atheist, but at least a deist. His commitment to a clockwork mechanistic-materialistic universe devoid of spirit is encapsulated by a famous but possibly apocryphal story: when Napoleon challenged him on where God fit in to his description of the universe, he stated “Je n’avais pas besoin de cette hypothèse-là.” (“I had no need of that hypothesis.”)

Laplace was also instrumental in developing the theory of Bayesian probability. It is in this aspect of his work that we meet his demon. Apart from many technical works on the subject, Laplace wrote a Philosophical Essay on Probabilities, the purpose of which was to present,

without the aid of analysis the principles and general results of this theory, applying them to the most important questions of life, which are indeed for the most part only problems of probability. Strictly speaking, it may even be said that nearly all of our knowledge is problematical; and in the small number of things which we are able to know with certainty, even in the mathematical sciences themselves, the principle means for ascertaining truth - induction, and analogy - are based on probabilities; and so the entire system of human knowledge is connected with the theory set forth in this essay.

And so, despite his determinism, Laplace is aware of the limits of human cognition. The purpose of probability, in his mind, is to fill those gaps. Certainly, there are classical deterministic systems that are chaotic and therefore not well-posed; while correct answers about the universe could be deduced from them in theory, in practice it is nearly impossible for mortal minds. Instead, this is the role of the demon:

Given for one instant an intelligence which could comprehend all the forces by which nature is animated and the respective situation of the beings who compose it — an intelligence sufficiently vast to submit these data to analysis — it would embrace in the same formula the movements of the greatest bodies of the universe and those of the lightest atom; for it, nothing would be uncertain and the future, as the past, would be present to its eyes.

This intelligence is what is now known as Laplace’s demon: a being smart enough and with enough knowledge to write the universe down as a well-posed differential equation, to solve it, and to play it forward and backward through time like an oscillating spring. To Laplace, we require probability theory only because of our feeble, mortal, ignorance. While chaotic systems may not yield useful predictions to us mortals, they may be useful for Laplace’s demon, whose infinite intelligence and knowledge could remove all uncertainty in the initial data and internal mechanisms and therefore yield the correct results.

The human mind offers, in the perfection which it has been able to give to astronomy, the feeble idea of this intelligence. Its discoveries in mechanics and geometry, added to that of universal gravity, have enabled it to comprehend in the same analytical expressions the past and future states of the system of the world. Applying the same method to some other objects of its knowledge, it has succeeded in referring to general laws observed phenomena, and in foreseeing those which given circumstances ought to produce. All these efforts in the search for truth tend to lead back continually to the vast intelligence which we have just mentioned, but from which it will always remain infinitely removed.

Thus, to Laplace, we are all just approximations of his demon.

Was Laplace correct in his radical determinism? Our current theories of the universe are indeterministic in a more fundamental way than classical chaos, and thus suggest that he was not. The laws of quantum mechanics (for instance, Heisenberg’s uncertainty principle) state that nature is fundamentally probabilistic in its dealings. Contrary to Einstein, it appears that God does in fact play dice; it would be impossible, even in principle, for Laplace’s demon to gain the knowledge of the position and momentum of each atom in the universe. According to the prevailing current philosophy, the universe is inherently indeterministic. There are, however, contemporary thinkers who seriously and credibly challenge this notion; for instance, I highly recommend this article by Nicolas Gisin. It is possible that Laplace’s demon still lurks on our horizon.

Maxwell’s Demon

Into the 19th century, it still looked as if Laplace’s dream of a mechanistic-deterministic universe, entirely understood by the laws of physics, was in reach. The mathematical physicist James Clerk Maxwell (1831–1879) made his illustrious career at this peak of classical physics, before relativity and the ultraviolet catastrophe made the world decidedly unclassical. Maxwell produced the most profound scientific revolution since the Newtonian one generations before, and paved the wave for the Einsteinian and Quantum revolutions to come. He came to realize that electricity, magnetism, and light were all instances of one unified phenomena, namely electromagnetic fields. It is to Maxwell we owe the governing partial differential equations for this vast set of phenomena which bear his name.

Maxwell did more for physics than just unify the electromagnetic phenomena. We are here more concerned with his work on thermodynamics, through which we get to meet his eponymous demon. In particular, Maxwell was concerned about the universal validity of the second law of thermodynamics, which states that the entropy of an isolated system can never decrease over time; it increases, or, at best remains constant if all processes are reversible. This law, being a general law, ought never be violated at any time in the universe; see the relevant quotation from Arthur Eddington’s The Nature of the Physical World (1927):

The law that entropy always increases holds, I think, the supreme position among the laws of Nature. If someone points out to you that your pet theory of the universe is in disagreement with Maxwell’s equations — then so much the worse for Maxwell’s equations. If it is found to be contradicted by observation — well, these experimentalists do bungle things sometimes. But if your theory is found to be against the second law of thermodynamics I can give you no hope; there is nothing for it but to collapse in deepest humiliation.

And yet it is just this law that Maxwell’s demon breaks. The demon first appears in a December 11, 1867 letter from Maxwell to Peter Tait, who had requested some insight for his own work on thermodynamics. Maxwell writes:

Any contributions I could make to that study are in the way of altering the point of view here and there for clearness or variety, and picking holes here and there to ensure strength and stability.
As for instance I think that you might make something of the theory of the absolute scale of temperature by reasoning pretty loud about it and paying it due honour at its entrance. To pick a hole — say in the 2nd law of ϴΔcs., that if two things are in contact the hotter cannot take heat from the colder without external agency.
Now let A and B be two vessels divided by a diaphragm and let them contain elastic molecules in a state of agitation which strike each other and the sides.
Let the number of particles be equal in A and B but let those in A have the greatest energy of motion. Then even if all the molecules in A have equal velocities, if oblique collisions occur between them their velocities will become unequal, and I have shown that there will be velocities of all magnitudes in A and the same in B, only the sum of the squares of the velocities is greater in A than in B.
When a molecule is reflected from the fixed diaphragm CD no work is lost or gained.
If the molecule instead of being reflected were allowed to go through a hole in CD no work would be lost or gained, only its energy would be transferred from the one vessel to the other.
Now conceive a finite being who knows the paths and velocities of all the molecules by simple inspection but who can do no work except open and close a hole in the diaphragm by means of a slide without mass.
Let him first observe the molecules in A and when he sees one coming the square of whose velocity is less than the mean sq. vel. of the molecules in B let him open the hole and let it go into B. Next let him watch for a molecule of B, the square of whose velocity is greater than the mean sq. vel. in A, and when it comes to the hole let him draw the slide and let it go into A, keeping the slide shut for all other molecules.
Then the number of molecules in A and B are the same as at first, but the energy in A is increased and that in B diminished, that is, the hot system has got hotter and the cold colder and yet no work has been done, only the intelligence of a very observant and neat-fingered being has been employed.
Or in short if heat is the motion of finite portions of matter and if we can apply tools to such portions of matter so as to deal with them separately, then we can take advantage of the different motion of different proportions to restore a uniformly hot system to unequal temperatures or to motions of large masses.
Only we can’t, not being clever enough.

Similarly, it is a consequence of the 2nd law of thermodynamics that an isolated gas with a hot part and a cold part will come to some temperature equilibrium at an intermittent temperature. Equivalently, you would never find the particles of a gas in a container organized so that all the fast-moving particles group together at one side. However, Maxwell’s demon is able to, by viewing the particles and quickly opening and closing a door in the middle of the container, to let only the fast-moving particles through, thus partitioning the gas and violated the 2nd law.

Maxwell, like Laplace and Descartes, did not originally call his demon a demon; this was apparently done by William Thomson (aka Lord Kelvin) in this 1879 note in Nature. In a subsequent undated letter to Tait, Maxwell writes:

Concerning Demons.
1. Who gave them this name? Thomson.
2. What were they by nature ? Very small BUT lively beings incapable of doing work but able to open and shut valves which move without friction or inertia.
3. What was their chief end ? To show that the 2nd Law of Thermodynamics has only a statistical certainty.
4. Is the production of an inequality of temperature their only occupation? No, for less intelligent demons can produce a difference in pressure as well as temperature by merely allowing all particles going in one direction while stopping all those going the other way. This reduces the demon to a valve. As such value him. Call him no more a demon but a valve like that of the hydraulic ram, suppose.

Whether “finite being,” demon, or valve, Maxwell’s thought experiment poses a serious challenge to the 2nd law of thermodynamics. Many rebuttals have been posed against Maxwell’s demon, but it remains a relevant idea in modern physics.

An important solution to the Maxwell demon problem has to do with information theory, as outlined in this lovely video by Eugene Khutoryansky. Basically, it boils down to the fact that on a fundamental level, information is physical. Where there is information stored, this ultimately increases the entropy of the surrounding environment. Another way of stating this is that information is entropy, in some sense. In order to perform her task, Maxwell’s demon must obtain information about the position and momentum of a large number of incoming particles. This information is stored (and subsequently erased) in the demon’s mind, increasing the entropy of the system in such a way that the 2nd law of thermodynamics isn’t violated, as long that the isolated system is analyzed in a way that includes the mind of the demon.

This solution is intimately related to Landauer’s principle, which gives us what can be called the information-energy equivalence; information storage implies energy storage, as information erasure implies energy dissipation. This principle has recently been extended to the mass-energy-information equivalence principle, applying Einstein’s famous equation E=mc² to Landauer’s principle. This principle states that information itself has mass! While this new idea is radical and unconfirmed, it is theoretically verifiable/falsifiable by experiment. If confirmed, it could even solve the outstanding problems that cosmologists have with the dark matter!

The fact that information is energy also leads us to notice that the demons of Laplace and Maxwell are conceptually related; Laplace’s demon would need to store the amount of information contained in the universe (and the associated mass/energy), plus that associated with his numerical algorithms, in order to be knowledgeable enough to do what Laplace invented him for! This may place a fundamental limitation on the kind of things we can calculate about the universe while remaining a part of the universe itself. But I digress.

Riemann’s Demon? A Mystery

I believe that we have exhausted all of the well-known demons present in the history of mathematical physics. However, in my readings I recently picked up the faintest traces of another. Some time in the year 1927 the illustrious poet Paul Valéry (1871–1954) wrote a letter to mathematician Jacques Hadamard, whom we mentioned in the introduction and here. Valéry writes:

Dans certaines recherches(que j’appellerai ‘philosophiques’ pour épargner notre temps) je me suis pris mille fois à souhaiter qu’un démon mathématique - non celui de Maxwell, mais celui de Riemann- apparût et m’aidât à me construire un schéma de mes idées simultanées.

In certain researches (which I shall call “philosophical” to spare our time) I have wished a thousand times that a mathematical demon — not the one of Maxwell, but the one of Riemann — appear and aid me in constructing a scheme of my simultaneous ideas.

Valéry is referring, of course, to Bernhard Reimann, who ranks among the very greatest mathematicians of all time. His achievements and contributions to modern mathematics are too vast to recount here, but we owe to Reimann the zeta function and the associated unproved Reimann hypothesis, along with groundbreaking advances in non-Euclidean geometry. However, unlike Descartes, Laplace, and Maxwell, there does not appear to be a well-know demon associated with his name.

What then did Valéry mean by “the demon of Reimann”? When I first came upon the letter to Hadamard, it was referenced in the biography Jacques Hadamard, a Universal Mathematician by Tatyana Shaposhnikova and Vladimir Maz’ya; the authors are themselves outstanding mathematicians with global renown. I had already begun formulating the ideas for this article, and was a naturally disturbed that I had not heard of this demon! Unable to contain my curiosity, I wrote the following email to Shaposhnikova and Maz’ya (who are a married couple), hoping that they could help me out:

Drs. Shaposhnikova and Maz’ya,

I hope this email finds you both safe and healthy. I apologize for the unsolicited email; I have a question that I hope either of you can answer.

I am currently reading your biography of Jacques Hadamard. In Chapter 4.2 you cite from a letter to Hadamard from Paul Valery:

“…I have wished a thousand times that a mathematical demon — not the one of Maxwell, but the one of Riemann — appear and aid me in constructing a scheme of my simultaneous ideas.”

I have long been fascinated with the so-called “demons” of the mathematicians and philosophers (Maxwell, Laplace, Descartes, etc.), but I am unable to find any other reference to a demon of Reimann! Do you know what Valery was referring to? Any description or references would be greatly appreciated.

Thank you for your time. Humbly yours,

Adam Taylor

To my delight, Dr. Maz’ya responded within a few hours:

Dear Adam,

We only remember a thought experiment by Maxwell (see the article Maxwell’s demon in Wikipedia). Perhaps other names are due to Valery’s imagination. In any case we are not aware of other demons.

With kind regards,

Vladimir Maz’ya

And so, the mystery still stands. Did Valéry have some knowledge about a personal or metaphorical demon associated with Reimann? Or was he, as Maz’ya suggests, just using imaginative language, as poets tend to do? Either way, I have been able to find no further reference to Riemann’s demon.

What is known is that Valéry read and understood deeply the scientific and mathematical works of Reimann, Poincaré, and others (see this 1960 article by Judith Robinson in the Modern Language Review). Perhaps, in his communication with Hadamard, he was simply using colorful language to describe the type of ethereal force of inspiration which is often required to forge works of genius. It is likely that Riemann’s “demon” is simply his otherworldly ability to discover and communicate groundbreaking mathematical ideas. If the reader is aware of any other meaning to Reimann’s demon, please let me know immediately.

A final aside: after nearly completing the writing of this article, I was made aware by a review in Nature of a brand new book entirely on the subject of scientific demons! While I have not yet read Jimena Canales’ work, it is going to the top of my list.