The Beautiful Life of John Forbes Nash, Jr.

This story is also available on Kindle!

“He was so incredibly himself” — Herta Newman

Mathematician John Forbes Nash, Jr (1928-2015). was born in Bluefield, West Virginia in 1928. He died in a car crash in New Jersey on the 23rd of May, 2015, on his way back home after receiving the renowned Abel Prize in Oslo a few days prior.

Popularized in the 2001 Academy Award winning movie A Beautiful Mind, Nash’s life story has since captured the fascination and imagination of the world. The goal of this article has been to celebrate that life. Here’s to John Forbes Nash, Jr., surely one of the most fascinating and brilliant people who has ever lived.

Early years (1928–45)

John Forbes Nash, Jr. was born on the 13th of June, 1928, in Bluefield, West Virginia. His father John Sr. was an electrical engineer for the Appalachian Electric Power Company. His mother, Margaret Virginia, had been a schoolteacher of Latin before she married. He had one younger sibling, his sister Martha who once recounted about her brother that “Nash was always different. My parents knew he was different. And they knew he was bright. He always wanted to do things his way”. Nash himself associated this trait with being picked on in school by his peers:

“One time, somebody suggested that I was a prodigy. Another time it was suggested that I should be called “bug brains,” because I had ideas, but they were sort of buggy or not perfectly sound.”

Of his influences, Nash in his Nobel autobiography describes reading a lot of books as a child, including Compton’s Pictured Encyclopedia “that I learned a lot from” (Kuhn et al, 2002 p. 6) and the later somewhat notorious Men of Mathematics (Bell, 1937). Early on, Nash also showed great interest in experimentation. By the time he was twelve, he had “turned his room into a laboratory. He tinkered with radios, fooled around with electrical gadgets, and did chemistry experiments. A neighbor recalled Johnny rigging the Nash telephone to ring with the receiver off” (Nasar, 1998). Even in high school, Nash remembers doing “electrical and chemistry experiments”. “At first when asked in school to prepare an essay about my career, I prepared one about a career as an electrical engineer like my father”.

To supplement their son’s education Nash’s parents arranged for him to take advanced mathematics courses at a local community college while he was still a senior in high school. That same year, he won a George Westinghouse Scholarship, one of ten awarded nationally. Following in is father’s footsteps, Nash in 1945 applied to the Carnegie Institute of Technology to pursue engineering.

At Carnegie Institute of Technology (1945–48)

One gets the sense that Nash felt out of place in Pittsburgh, where he for four years attended college at the Carnegie Institute of Technology (now Carnegie Mellon University) as an undergraduate. First enrolled in a chemical engineering program (later switching to chemistry) Nash spent much of his time there rebelling against the regimentation of the program and the lack of mathematical rigour in his courses. A born researcher, he would object to the notion that performance was measured “not by how well one could think, but of how well one could handle a pipette and perform titration in the laboratory” (Nasar, 1998). He was so bored during his summer job at the Westinghouse Lab that he spent most of the two months there making and polishing a brass egg in the lab’s machine shop.

However, as he returned for his sophomore year, Nash discovered that a brilliant group of new researchers had joined the university faculty, including physicists John Synge (1871-1909), Richard Duffin (1909-96), and mathematicians Raoul Bott (1923-2005) and Alexander Weinstein (1897-1979). From the start, Nash caught their attention with his brilliance. They eventually urged him to switch from chemistry to mathematics and seriously consider an academic career (Nasar, 1998).

“In the fall of 1947, Professor Richard Duffin stood at the board frowning. “He was intimately familiar with Hilbert spaces, but he had prepared his lecture too hastily, had wandered down a cul de sac in the course of his proof, and was hopelessly stuck. It happened all the time.” The five students in the advanced graduate class were getting restive. After a few moments, everybody, including the professor, turned toward the gawky undergraduate in the back, squirming in his seat. “Okay John, you go to the board. See if you can get me out of trouble.” Nash leaped up and strode to the blackboard.”

— Excerpt, A Beautiful Mind by Sylvia Nasar (1998)

By the middle of his second year Nash was concentrating almost exclusively on mathematics.

“I shifted again and became officially a student of mathematics. […] In the end I had learned and progressed so much in mathematics that they gave me an M. S. in addition to my B. S. when I graduated.”—Nash in 1994

By the spring of 1948, in what would have been his junior year, Nash had been accepted to Harvard, Princeton, Chicago and Michigan University, the top four graduate-level math programs in the U.S. at the time. From his biographer Nasar’s interviews we know that at Carnegie, both Duffin and Synge were pushing Nash to choose Princeton. Its “hothouse milieu of pure mathematicians (topologists, algebraists, number theorists)” likely seemed like the perfect place for “a young Gauss”, as one of them called him (Nasar, 1998). On the Princeton side, the chairman of the mathematics department Solomon Lefschetz (1884-1972) was equally eager in persuading Nash, eventually offering a John S. Kennedy Fellowship of $1,150 per year.

“We like to catch promising men when they are young and open-minded”

- Excerpt from a letter from Solomon Lefschetz to Nash

Although Harvard was his first choice (for its reputation, social status and faculty), Nash’s mediocre performance on the esteemed Putnam Competition had lead Harvard to offer slightly less money than Princeton. Princeton’s proximity to his family in Bluefield was an additional consideration, according to his Nobel autobiography. These factors, adding to the encouragement both of his supervisors at Carnegie and Lefschetz’s personal appeal, Nash eventually decided on Princeton, and left for New Jersey in the summer of 1948.

At Princeton University (1948–51)

Nash entered graduate school when he was 20 years old, three years after leaving Bluefield. At the time, Princeton’s math department was filled with brilliant minds, lead by Lefschetz who jointly with Ralph Fox (1913-73) and Norman Steenrod (1910-71) headed research on topology, first in the country. Emil Artin (1898-1962) lead algebra. Student of Lefschetz, Albert W. Tucker (1905-95) lead game theory which at that point was a newly established discipline entirely, invigorated by the publication of the book Theory of Games and Economic Behavior by John von Neumann (1903-57) and economist Oskar Morgenstern (1902-78) in 1944.

Princeton’s math department, housed in Fine Hall in the 40s and 50s has since become somewhat legendary in mathematical circles. As Nasar recounted in 1998, “Fine Hall is, I believe, the most luxurious building ever devoted to mathematics, [according to] one European émigré. [..] A country club for math, where you could take a bath.”

“Its cornerstone contains a lead box with copies of works by Princeton mathematicians and the tools of the trade — two pencils, one piece of chalk, and, of course, an eraser. Designed by Oswald Veblen [...] it was meant to be a sanctuary that mathematicians would be ‘loath to leave’. The dim stone corridors that circled the structure were perfect for both solitary pacing and “mathematical socializing. The nine “studies” — not offices! — for senior professors had carved paneling, hidden file cabinets, blackboards that opened like altars, oriental carpets, and massive, overstuffed furniture. [...] Each office was equipped with a telephone and each lavatory with a reading light.” Its well-stocked third-floor library, the richest collection of mathematical journals and books in the world, was open twenty-four hours a day. Mathematicians with a fondness for tennis (the courts were nearby) didn’t have to go home before returning to their offices — there was a locker room with showers.”

— Excerpt, A Beautiful Mind by Sylvia Nasar (1998)

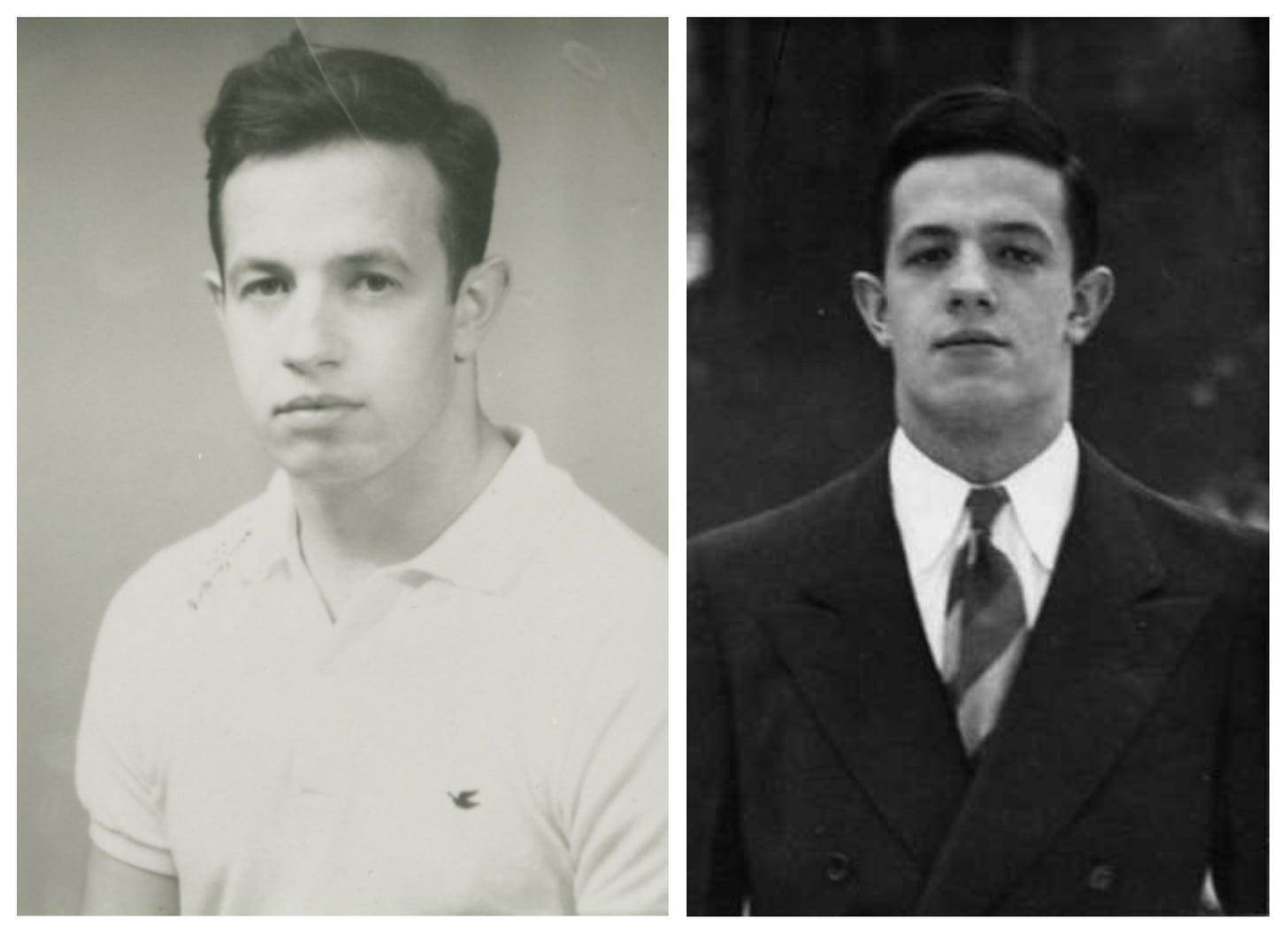
Nash was part of the clique of mathematicians and graduate students advancing the nascent discipline of game theory under Tucker, in the purest mathematical sense (i.e. largely uninterested in relating their research to applications in the real world). According to economist and personal friend of Nash, Martin Shubik (1926-2018):

"The graduate students and faculty in the mathematics department interested in game theory were both blissfully unaware of the attitude in the economics department, and even if they had known it, they would not have cared.. The contrast of attitudes between the economics department and the mathematics department was stamped on my mind soon after arriving at Princeton. The former projected an atmosphere of dull business-as-usal conservatism of a middle league conventional Ph.D. factory; there were some stars but no sense of excitement or challenge. The latter was electric with ideas and the sheer joy of the hunt. Psychologically they dwelt on different planets. If a stray ten-year-old with bare feed, no tie, torn blue jeans and an interesting theorem had walked into Fine Hall at tea time, someone would have listened. When von Neumann gave his seminar on his growth model, with few exceptions, the serried ranks of Princeton Economics cold scare forbear to yawn."

— Excerpt, Finding Equilibrium by Düppe and Weintraub (2014 p. 94)

The head of the group, Tucker, would go on to supervise virtually all of the future top game theorists at Princeton, including David Gale (1921-2008) and 2012 Nobel laureate Lloyd Shapley (1923-2016), in addition to, of course, Nash.

Nash the Game (Hex)

The story of Nash’s most famous result, as often is the case, departs not from an interest in its application or streams of theoretical research, but rather as the consequence of experimentation. Read the story here:

Privatdozent 🇺🇦John F. Nash Jr.'s Game of "Hex" (1949)By the late 1940s, the favorite past-time of faculty and graduate students in Fine Hall at Princeton University was board games, including the famous Go and Chess, as well as the less famous Kriegspiel. During this time, ‘beautiful mind’ John Forbes Nash Jr…Read more2 years ago · 7 likes · Jørgen Veisdal

Nash’s Research (1948–58)

“In the taxonomy of mathematicians, there are problem solvers and theoreticians, and, by temperament, Nash belonged to the first group”. — Nasar (1998)

As a consequence of the mental illness that would later consume him, Nash’s prime research career was remarkably short, essentially only spanning nine years from his arrival at Princeton in 1948 to his diagnosis in 1958. Raoul Bott at Carnegie said this about Nash’s interests as a graduate student:

“Nash liked very general problems. He wasn’t all that good at solving cute little puzzles. He was a much more dreamy person. He’d think a long time. Sometimes you could see him thinking. Others would be sitting there with their nose in a book.”

Of his own graduate research, Nash himself stated in his Nobel autobiography that “As a graduate student I studied mathematics fairly broadly and was fortunate enough, besides developing the idea which led to “Non-Cooperative Games”, also to make a nice discovery relating to manifolds and real algebraic varieties. So I was prepared actually for the possibility that the game theory work would not be regarded as acceptable as a thesis in the mathematics department and then that I could realize the objective of a Ph.D. thesis with the other results”.

Nash ultimately didn’t need to “realize the objective of a Ph.D. thesis with other results”. He earned his Ph.D. in mathematics from Princeton in 1950 at the age of 22. His 28-page dissertation was entitled Non-cooperative Games (1950). It is available here. Written under the supervision of Tucker, the main result of the paper was the derivation, definition and description of the properties of the Nash equilibrium.

The Bargaining Problem (1949)

Nash’s first journal paper (written prior to his work on the Nash equilibrium) — also in game theory — regarded the classic economic problem of bargaining. The problem had previously been investigated by a number of scholars, including Antoine Augustin Cournot (1801-77), Arthur L. Bowley (1869-1957) and William Fellner (1905-83), among others (Nash, 1950a). Read more about the problem and Nash’s solution here:

Privatdozent 🇺🇦Nash's Bargaining Problem (1950)Just before John Nash made his breakthrough discovery of the Nash equilibrium, he wrote his first paper on another problem later to be known as Nash’s bargaining game. Nash’s bargaining game is a study of cooperative bargaining and the demonstration of a unique solution to a two-person bargaining problem (Nash, 1950). Stated simply, the setup for the bargining problem is as follows…Read more2 years ago · 11 likes · Jørgen Veisdal

Meeting John von Neumann

Although somewhat in opposition to von Neumann and Morgenstern’s work on cooperative game theory, Nash’s results establishing a foundation for non-cooperativegame theory clearly had its origins in the formers’ work. Indeed, illustrative of this, in 1978 Nash was awarded the John von Neumann Theory Prize for his discovery of the Nash equilibrium.

Only one documented account of communication between Nash on von Neumann can now be found, although there were surely many more now lost to time. According to Nasar, Nash went to talk to von Neumann a few days after he passed his general examination at Princeton in 1949, prior to his work on the Nash equilibrium. As she writes:

“He wanted, he had told the secretary cockily, to discuss an idea that might be of interest to Professor von Neumann. It was a rather audacious thing for a graduate student to do. [...] But it was typical of Nash, who had gone to see Einstein the year before with the germ of an idea. [...] He listened carefully, with his head cocked slightly to one side and his fingers tapping. Nash started to describe the proof he had in mind for an equilibrium in games of more than two players. But before he had gotten out more than a few disjointed sentences, von Neumann interrupted, jumped ahead to the yet unstated conclusion of Nash’s argument, and said abruptly, “That’s trivial, you know. That’s just a fixed point theorem.”

— Excerpt, A Beautiful Mind by Sylvia Nasar (1998)

von Neumann, in other words, did not see the value in Nash’s bargaining result. Nash himself however would later defend the great man’s reaction in a letter to Robert Leonard, stating, characteristically analytically, “I was playing a non-cooperative game in relation to von Neumann rather than simply seeking to join his coalition. And of course, it was psychologically natural for him not to be entirely pleased by a rival theoretical approach”. Both von Neumann and Morgenstern ultimately did however provide Nash with valuable guidance, and in the published version Nash makes sure to acknowledge the role of both, writing “The author wishes to acknowledge the assistance of Professors von Neumann and Morgenstern who read the original form of the paper and gave helpful advice as to the presentation.”

The Nash Equilibrium (1950)

A few days after his meeting with von Neumann, Nash reportedly again “accosted” fellow student David Gale on campus:

“I think I’ve found a way to generalize von Neumann’s min-max theorem,” he blurted out. “The fundamental idea is that in a two-person zero-sum solution, the best strategy for both is … The whole theory is built on it. And it works with any number of people and doesn’t have to be a zero-sum game!”

Characteristically, as Nasar writes, Gale was less enchanted by the possible applications of the work than the mathematics, stating in 1995 that “The mathematics was so beautiful. It was so right mathematically.”

“Gale realized that Nash’s idea applied to a far broader class of real-world situations than von Neumann’s notion of zero-sum games. “He had a concept that generalized to disarmament”

— Excerpt, A Beautiful Mind by Sylvia Nasar (1998)

Gale also helped Nash claim credit for the result as soon as possible by drafting a note to the National Academy of Sciences. Lefschetz submitted the note on their behalf, and the result appeared in less than a single page entitled ‘Equilibrium Points in N-Person Games’ in the 36th volume of the Proceedings of the National Academy of Sciences in January of 1950.

The result, later to be known as the Nash equilibrium is now typically formally defined as follows:

Definition of a Nash equilibrium

Let (S,f) be a game with u players Sᵢ is the set of strategies for player i, S = S₁ x S₂ x ... x Sᵤ is the set of strategy profiles and f(x) = (f₁(x),...,fᵤ(x)) is its payoff function evaluated at x ∈ S. Let xᵢ be a strategy profile of player i and x₋ᵢ be a strategy profile of all players except player i.

When each player i ∈ {1,...,u} chooses a strategy xᵢ, resulting in a strategy profile x = (x₁,...,xᵤ) then player i obtains payoff fᵢ(x). Note that the payoff depends on the strategy profile chosen, i.e. on the strategy chosen by player i as well as the strategies chosen by all the other players.

A strategy profile x* ∈ S is a Nash equilibrium if no unilateral definition in strategy by any single player is profitable for that player, that is

∀i,xᵢ ∈ Sᵢ : fᵢ(x*ᵢ, x*₋ᵢ) ≥ fᵢ(xᵢ,x*₋ᵢ)

Informally, the theorem states:

A strategy profile is a Nash equilibrium if no player can do better by unilaterally changing his or her strategy.

That is, in a two-person game, a pair of strategies constitute a Nash equilibrium if player A’s choice is optimal, given player B’s choice, and B’s choice is optimal given player A’s choice. No player can singlehandedly change their strategy in order to obtain a more optimal result. Crucially, neither player knows what strategy the other will choose, but acts solely on the basis of their own interests, given their knowledge of other players’ interests. The finding generalizes to n players.

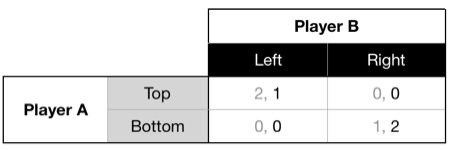
In the table above, the strategies and payoffs of a two-person game are shown. Player A can choose between the strategies Top and Bottom. If player A chooses Top, he will receive a payoff of 2 if player B chooses Left and 0 if player B plays Right. If player A chooses Bottom, he will receive a payoff 0 if player B plays Left, and 1 if player B plays Right. Thus, player A’s optimal choice depends on what he thinks player B will do (Varian, 2006 p. 506).

In the table above, the strategy set (Top, Left) is a Nash equilibrium. To show it, note that if A chooses Top, then the best thing for B to do is to choose Left, since the payoff for B from choosing Left is 1 and from choosing Right is 0. If B chooses Left, then the best thing for A to do is to choose Top since then A will get a payoff of 2 rather than of 0. Thus, if A chooses Top, the optimal strategy for B is to choose Left ; and if B chooses Left, then the optimal strategy for A is to choose Top. So, we have a Nash equilibrium: each person is playing their optimal strategy, given the other player’s strategies.

Proofs of the Nash Equilibrium

As mentioned, Nash’s thesis proof (1950c) used Brouwer’s fixed-point theorem. A version of this “clumsy, if totally original” proof (Kuhn et al, 2002), a version can be found here. Awarding credit to Gale, Nash later published a simpler proof of the same result, using the Kakutani fixed-point theorem. It goes as follows:

Proof of the existence of Nash Equilibria using the Kakutani fixed-point theorem (Nash, 1951)

To prove the existence of a Nash Equilibrium (NE), let rᵢ(σ₋ᵢ) be the best response of player i to the strategies of all other players.

rᵢ(σ₋ᵢ) = arg max uᵢ(σᵢ, σ₋ᵢ)

Here, σ ∈ Σ where Σᵢ x Σ₋ᵢ is a mixed strategy profile in the set of all mixed strategies and uᵢ is the payoff function for player i. Define a set valued function r: Σ → 2^Σ such that r = (rᵢ(σ₋ᵢ), r₋ᵢ(σ₋ᵢ). Proving the existence of a Nash equilibrium is equivalent to showing that r has a fixed point.

Kakutani's fixed point theorem guarentees the existence of a fixed point if the following four conditions are satisfied:
1. Σ is compact, convex and non-empty
2. r(σ) is nonempty
3. r(σ) is upper hemicontinuous
4. r(σ) is convex

Condition 1 is satisfied from the fact that Σ is a simplex and thus compact. Convexity follows from players' abilities to mix strategies. Σ is non-empty as long as players have strategies.

Condition 2. and 3. are satisfied by way of Berge's maximum theorem. Because uᵢ is continuous and compact, r(σ) is non-empty and upper hemicontinuous. 

Condition 4 is satisfied as a result of mixed strategies. Suppose σᵢ, σᵢ' ∈ r(σ₋ᵢ), then λσᵢ + (1 - λ)σᵢ' ∈ r(σ₋ᵢ), i.e. if two strategies maximize payoffs, then a mix between two strategies will yield the same payoff. 

Therefore, there exists a fixed point in r and a Nash equilibrium.

Interpretations

Nash in his thesis proposed two ways of thinking about his equilibrium concept: one based on rationality and one based on statistical populations. In the rational interpretation, players are perceived as rational and they have complete information about the structure of the game, including all of the players’ preferences regarding possible outcomes, where this information is common knowledge. Since all players have complete information about each others’ strategic alternatives and preferences, they can also compute each other’s optimal choice of strategy for each set of expectations. If all of the players expect the same Nash equilibrium, and the game is played only once, then there are no incentives for anyone to change their strategies. In the interpretation according to statistical populations, Nash states that “[i]t is unnecessary to assume that the participants have full knowledge of the total structure of the game, or the ability and inclination to go through any complex reasoning processes”. This because “What is assumed is that there is a population of participants for each position in the game, which will be played throughout time by participants drawn at random from the different populations. If there is a stable average frequency with which each pure strategy is employed by the average member of the appropriate population, then this stable average frequency constitutes a mixed strategy Nash equilibrium.” (Nash, 1950c). 

As Kuhn would later write:

"The Nobel selection committee apparently took the two interpretations that are contained in the thesis seriously. The rational interpretation could have been argued by Cournot, but the statistical interpretation, which is so important for biological games, is wholly original. Although the nature of non-cooperative games is explained in all three of these papers, only the thesis contains an exposition of these two interpretations. When asked at the Nobel seminar why the interpretations were not included in the Annals paper. Nash responded, "I don't know whether it was just pruned down in style for the Annals of Mathematics."

- Excerpt, The Essential John Nash by Kuhn et al (2002)

Journal papers

Nash’s thesis would spawn three journal papers. The three articles contain three different proofs of the existence of Nash equilibria. The first, entitled ‘Equilibrium Points in N-person Games’ (1950b) is the note Nash and Gale drafted for the Proceedings of the National Academy of Sciences. The second, called ‘Non-Cooperative Games’ (1951) was published in the Annals of Mathematics Vol. 54 (2). In ‘Two-person Cooperative Games’ (1953), published in Econometrica 21, Nash extends his work on the bargaining problem (Nash, 1950a) to a wider class of situations in which threats can a play a role (Kuhn et al, 2002).

Applications

Barring its mathematical nature and interesting theoretical implications for economics, the Nash equilibrium is celebrated mostly due to its many real-world applications. Nasar (1998) highlights its role in auction design in the 1990s. Other applications typically highlighted are war and arms races, conflict mitigation, cooperation, risk avoidance, in the adoption of technical standards and analysis of bank runs and currency crises, traffic flow, environmental legislation and in analyzing evolutionary processes such as natural selection in evolutionary biology.

For those interested, Nash’s work in game theory was beautifully collected and organized in this article prepared for the Nobel Committee by his friend, mathematician Harold Kuhn (1925-2014) in 1994. Fields medal winner John Milnor(1931-) in 1998 also wrote notice for the American Mathematical Society listing Nash’s total of 21 publications.

Other results

Although—by far—recognized most widely for the work he did in game theory, Nash in the 1950s however also wrote groundbreaking papers on real algebraic geometry, topology and partial differential equations. Indeed, he won the Abel Prize in 2015 for his work on the latter, alongside Louis Nirenberg (1926-2020), considered one of the most outstanding pure mathematicians of the 20th century. For pure mathematicians, this subsequent work of Nash’s, which was accomplished just before his illness set in, is considered far more rich and important.

The bibliography assembled by Milnor in 1998 lists 21 publications to Nash’s name. However, excluding his work in game theory and economics, as well as conference papers and book chapters, Nash’s original work in pure mathematics is essentially contained in four journal papers, written between 1952-58:

• Nash, J.F. 1952. Real Algebraic Manifolds. Annals of Mathematics 56(3), pp. 405-421.
• Nash, J.F. 1954. C¹ Isometric Imbeddings. Annals of Mathematics 60(3), pp. 386-396.
• Nash, J.F. 1956. The Imbedding Problem for Riemannian Manifolds. Annals of Mathematics 61(3), pp. 20-63.
• Nash, J.F. 1958. Continuity of Solutions of Parabolic and Elliptic Equations. American Journal of Mathematics 80(4). pp. 931–954.

A discussion of these papers and how Nash arrived at their results can be found in the following previous newsletter:

PrivatdozentJohn F. Nash's Work in Pure MathematicsIt’s fair to say that John F. Nash Jr. (1928-2015) didn’t earn much respect from pure mathematicians for his work in game theory. To them, his use of L.E.J. Brouwer (1881-1966) and Shizuo Kakutani (1911-2004)’s fixed-point theorems to show the existence of Nash equilibria in finite games with mixed strategies was and perhaps still is merely considered…Read more2 years ago · 9 likes · Jørgen Veisdal

Marvin Minsky’s Ph.D. problem

“He was not a normal human being. He was pathologically logical.” — Marvin Minsky

Nasar fleetingly mentions Marvin Minsky (1927-2016)’s presence at Princeton in the 1950s a few times in her book. In speaking with her, Minsky draws a parallel between the personalities of himself and Nash, stating “We shared a similarly cynical view of the world. We’d think of a mathematical reason for why something was the way it was. We thought of radical, mathematical solutions to social problems. At one point, Nash suggested a complete transfusion for something. If there was a problem, we were good at finding a really ridiculously extreme solution.”

According to Minsky himself, he was having trouble proving “what can be accomplished by loops of neurons that are arranged in circular pathways, so that if you put a certain pattern in it will sort of echo around and under some conditions, the information that you originally put into such a loop will be gradually destroyed and the pulses will be come equally spaced.”

Following a brief moment of reflection, Nash provided him with the necessary solution. “Why don’t you expand that into a Fourier series?”.

“After a couple of hours I figured out what that would mean and I did it, and I proved this theorem.” — Marvin Minsky

Meeting Albert Einstein

Although highlighted here and in most other narrations of Nash’s work, game theory barely scratched the surface of his interests in mathematics at Princeton. As such, Nasar writes that “it was a measure of Nash’ bravura and the power of his fantasy” that he was not merely satisfied to walk by fellow Princetonian Albert Einstein (1879-1955) as he was commuting between his house and his office at The Institute for Advanced Study. Nash requested an audience with him.

A fresh new graduate student at Princeton, Nash made an appointment “discuss an idea with Professor Einstein” in his office in Fuld Hall. As Nasar writes, he was ushered into the messy, large and airy room with a bay window by Einstein’s “Martian” assistant John Kemeny (1926-92), the later inventor of BASIC. Nasar writes “Einstein’s handshake, which ended with a twist, was remarkably firm, and he showed Nash to a large wooden meeting table on the far side of the office”.

“As Einstein twirled the curls on the back of his head with his finger while sucking on a tobaccoless pipe, Nash matter-a-factly laid out his ideas about “gravity, friction and radiation”. The idea he had been thinking of revolved around the friction particles like photon experiences as it is moved through space by its fluctuating gravitational field interacting with other gravitational fields. Nash, Einstein and Kemeny discussed the topic for close to an hour, at the end of which Einstein ended concluding to the tall, broadshouldered 20-year-old that “You had better study some more physics, young man”

- Excerpt, A Beautiful Mind by Sylvia Nasar (1998)

At MIT

Following his graduation from Princeton, both Chicago University and the Massachusetts Institute of Technology (MIT) were interested in hiring Nash, then 23 years old. Nash chose the latter, beginning work as a C. L. E. Moore instructor in MIT’s math department in June of 1951. Anecdotes about Nash as an instructor at MIT abound. According to Nasar, on one occasion Nash was confronted by a grader on one of his exams for putting the following problem on a test:

If you make up a bunch of fractions of pi 3.141592…. If you start from the decimal point, take the first digit, and place decimal point to the left, you get .1
Then take the next 2 digits .41
Then take the next 3 digits .592
And so on and so on.
You get a sequence of fractions between 0 and 1.
What are the limit points of this set of numbers?

- Excerpt, "A Beautiful Mind" by Sylvia Nasar (1998)

As the story goes, the problem had never been solved before. Nash defended his putting it on an exam by stating “Maybe, if people didn’t realize that the problem was ‘hard,’ they could solve it” (Nasar, 1998).

Mental Illness (1959–80s)

“These ideas came to me the same way my mathematical ideas did. So I believed them” — Nash

I resist the temptation of summarizing Nash’s life story as that of a highly intelligent paranoid schizophrenic. Illness aside, Nash was primarily a mathematician, a highly cited researcher and eventually both a Nobel Laureate and an Abel Prize recipient. However, in the interest of completeness, I will recount some anecdotes which may be of relevance to those interested in the properties of an exceptional mind such as Nash’s.

Nash’s mental illness first manifested as paranoia. Alicia lated described his behavior as erratic. However, according to Nasar, despite many eccentric appearances in and around MIT’s math department, Nash’s mental difficulties did not immediately take notice among his peers. As Bott recalled, “his conversation always mixed mathematics and myth”. “In his game theory course, Nash behaved like his usual self, according to students who were in the class. […] He gave a midterm without announcing it in advance. He also paced a great deal and sometimes fell into reveries in the middle of lecturing or answering a student’s question.” As he walked across the Charles river with two TAs, “Nash embarked on a lengthy monologue that was difficult to follow. […] It concerned threats to world peace and calls for world government. Nash seemed to be […] hinting that he had been asked to play some extraordinary role” (Nasar, 1996).

The number theorist Atle Selberg (1917-2007) recounted to Nasar about a seminar in Cambridge, where Nash was asking “some questions I thought were in a sense, to my way of thinking, somewhat inappropriate to the subject. He seemed to see something quite different than what I had intended…. [His] questions were formulated as if I had some hidden, not fully disclosed, agenda that he wanted to discover. The lecture was about the rigidity of several locally symmetric spaces. Nash was in the audience. He asked some questions that seemed to imply I had a hidden, secret motive. He suspected it had something to do with the Riemann Hypothesis, which of course it did not. I was rather taken aback. This was something that had nothing to do whatsoever [with the Riemann Hypothesis].”

Nash would be hospitalized for the first time in 1959. From Nasar’s interviews, she describes that the commitment was likely arranged by MIT’s psychiatric service, probably in consultation with the president of the university in conjunction with William Ted Martin (1911-2004) and Norman Levinson (1912-75). In 1961, he was admitted to the New Jersey State Hospital at Trenton where he received both antipsychotic medications and insulin shock therapy. Over the next nine years, he would spend periods in and out of psychiatric hospitals, bouncing between periods of lucidity and paranoia. After 1970, he was never committed to a hospital again, famously refusing to take medications against schizophrenia for the rest of his life. According to Nash himself:

“After my return to the dream-like delusional hypotheses in the later 60s I became a person of delusionally influenced thinking but of relatively moderate behavior and thus tended to avoid hospitalization and the direct attention of psychiatrists.”

Nash’s View of Remission

“I don’t really remember the chronology very well, exactly when I moved from one type of thinking to another. I began arguing with the concept of the voices. And ultimately I began rejecting them and deciding not to listen”

Nash, by his own account and will, indeed stopped taking the medication he was prescribed sometime in the 1970s, stating “I began to realize that I would not be getting out of the hospital unless I conformed and behave normally, and so in part I would do that — as if I would be sweeping the delusions under a rug.” When asked how he got better, according to Kuhn, Nash said “I willed it” (implying that he chose to ignore his delusions and actively work to think rationally).

“Gradually I began to intellectually reject some of the delusionally influenced lines of thinking which had been characteristic of my orientation. This began, most recognizably, with the rejection of politically oriented thinking as essentially a hopeless waste of intellectual effort.”

By the 1980s, Nash was back in Princeton working on mathematics and auditing classes. During this period, among undergraduates especially, he would become known as “The Phantom of Fine Hall”, a “shadowy figure who would scribble arcane equations of blakboards in the middle of the night” (Kwon, 2010).

The Nobel Prize (1994)

“Jubilant! We danced around our kitchen!” — Herta Newman

Several weeks before the 1994 Nobel prize in economics was announced on Oct. 11, two mathematicians — Harold W. Kuhn and John Forbes Nash Jr. — visited their old teacher, Albert W. Tucker, now almost 90 and bedridden, at Meadow Lakes, a nursing home near here. Mr. Nash hadn’t spoken with his mentor in several years. Their hour-long conversation, from which Mr. Kuhn excused himself, concerned number theory.

When Mr. Nash stepped out of the room, Mr. Kuhn returned to tell Mr. Tucker a stunning secret: Unbeknownst to Mr. Nash, the Royal Swedish Academy intended to grant Mr. Nash a Nobel Prize for work he had done as the old man’s student in 1949, work that turned out to have revolutionary implications for economics. The award was a miracle. — Nasar, 1994.

On the 11th of October 1994, Sveriges Riksbank Prize in Economic Sciences in Memory of Alfred Nobel announced that the 1994 Nobel Prize in Economics would be awarded to Dr. John Forbes Nash, Jr:

The Royal Swedish Academy of Sciences has decided to award the Bank of Sweden Prize in Economic Sciences in Memory of Alfred Nobel, 1994, jointly to:

Professor John C. Harsanyi, University of California, Berkeley
Dr. John F. Nash, Princeton University
Professor Reinhard Selten, Rheinische Friedrich-Wilhelms-Universität

For their pioneering analysis of equilibria in the theory of non-cooperative games.

The press release distinguishes non-cooperate game theory as separate from the pioneering early work of von Neumann and Morgenstern. About Nash’s research, the committee wrote:

John F. Nash introduced the distinction between cooperative games, in which binding agreements can be made, and non-cooperative games, where binding agreements are not feasible. Nash developed an equilibrium concept for non-cooperative games that later came to be called Nash equilibrium.

Later life (1980s–2015)

“He shined very brightly as a young man. Then he had his illness, and is now a very pleasant, accomplished gentleman. It feels right, somehow. “ — Erhan Cinlar (2002)

Nash had a son with his first girlfriend, a nurse named Eleanor Stier (1921–2005) in 1953. The child was named John David Stier and was born on the 19th of June. While still a graduate student at Princeton, Nash met Alicia Lardé. The two married in February 1957 and had a son, John Charles Martin Nash, who later earned a Ph.D. in mathematics from Rutgers University, is a chess Grand Master and also suffers from schizophrenia. In the midst of his illness, Alicia divorced him in 1963 but they continued living together. They re-married 38 years later, in 2001.

A Beautiful Mind

Much of the credit for the recognition and documentation of Nash’s life and career (and indeed this essay) goes to his biographer Sylvia Nasar. Her book A Beautiful Mind was released in 1998, became a New York Times Best Seller and in the same year won the National Book Critics Circle award for biography and was a finalist for the Pulitzer Prize.

An adaptation of the book was later written by Akiva Goldsman. The movie — directed by Ron Howard starring Russell Crowe as Nash — was released in 2001. It went on to gross over $313 million worldwide and won four academy awards, including for Best Picture, Best Director and Best Adapted Screenplay.

Ron Howard thanked Nash and Alicia both during his Academy Awards acceptance speech. Upon release of the film, Nash’s biographer Sylvia Nasar appeared on the Charlie Rose Show to recount Nash’s story in a segment entitled Schizophrenia and Genius.

Around the time of the release of the movie, a segment about Nash was also featured on CBS’ 60 Minutes. An essay about the segment was published following Nash’s death in 2015. Also around the same time as the release of the movie, Nash’s longtime friend and fellow Princeton mathematician Harold W. Kuhn co-edited a biography of Nash’s life in 2002 entitled The Essential John Nash.

The Abel Prize

On the 25th of March 2015, the Norwegian Academy of Sciences and Letters announced that the 2015 Abel Prize was to be awarded to John Nash and Louis Nirenberg for their “striking and seminal contributions to the theory of nonlinear partial differential equations and its applications to geometric analysis.”

Death and Beyond (2015)

John and Alicia were killed in a car crash on the 23rd of May 2015. They were traveling back from Newark airport to Princeton after their trip to Oslo where Nash received his Abel Prize. According to New Jersey State Police, the taxi they were riding in was traveling southbound in the left lane on the New Jersey Turnpike when the driver lost control while trying to pass another vehicle. The taxi crashed into the guardrail and then into another car in the right lane (USA Today, 2015). Neither John, nor Alicia were wearing seatbelts.

Press release from Princeton University.

Nash’s Nobel medal

On the 30th of August 2016 it was made public that one of Nash’s surviving sons, John Stier, was putting Nash’s Nobel Medal up for auction at Sotheby’s. The medal did not sell. However, the medal was later sold by Christies for $735,000 in part to benefit the John C. M. Nash Trust.

Open Problems in Mathematics

In his later years, Nash co-edited a book of essays on the current status of the solutions to some of the most essential open problems in pure mathematics with Michael Th. Rassias. The book is entitled Open Problems in Mathematics. Unfortunately, Nash did not live to see the publication of the book, which was published in 2016.

Unpublished Works

Nash published notes, scans and photographs from his work, trips and lectures on his personal website at Princeton. It is still accessible here.

Thank you for subscribing to the Privatdozent newsletter.

Sincerely,

Jørgen.

Related Privatdozent Essays

• Nash's Bargaining Problem (1950), December 3rd 2021
• John F. Nash Jr.’s Game of “Hex”, October 10th 2021
• John von Neumann’s Minimax Theorem (1928), March 26th 2021
• Kurt Gödel’s Brilliant Madness, June 21st 2021
• The Anarchist Abstractionist — Who was Alexander Grothendieck?, September 17th 2021
• The Mathematics of Ted Kaczynski, April 18th 2021

About

The Privatdozent newsletter currently goes out to 8,527 subscribers via Substack.

References

• Düppe, T. & Weintraub, E. R. 2014. Finding Equilibrium: Arrow, Debrau, McKenzie and the Problem of Scientific Credit. Princeton University Press.
• Kwon, Ha Kyung 2010. “Nash GS ’50: ‘The Phantom of Fine Hall’”. The Daily Princetonian. Retrieved May 6, 2014.
• Nasar, S. 1994. The Lost Years of a Nobel Laureate. The New York Times.
• Nasar, S. 1998. A Beautiful Mind. Simon & Schuster
• Nash, J.F. 2002. The Essential John Nash. Princeton University Press.
• Nash, J.F. and Rassias, M.T. 2016. Open Problems in Mathematics. Springer International Publishing.
• Nash, J.F. 1995. John Forbes Nash Jr. — Biographical. NobelPrize.org. Nobel Media AB 2019. <https://www.nobelprize.org/prizes/economic-sciences/1994/nash/biographical/>
• Nash, J.F. 1950a. “The Bargaining Problem.” Econometrica 18: 155–162.
• Nash, J.F. 1950b. “Equilibrium Points in N-Person Games.” Proceedings of the National Academy of Sciences 36: 48–49.
• Nash, J.F. 1950c. “Non-cooperative Games.” Ph.D. diss., Mathematics Department, Princeton University.
• Nash, J.F. 1951. “Non-cooperative Games.” Annals of Mathematics 54: 286–295.
• Nash, J.F. 1953. “Two-Person Cooperative Games.” Econometrica 21: 128–140.
  Schouten, F. (1995). ‘A Beautiful Mind’ mathematician John Nash, wife killed in crash. USA Today. Available at: <https://eu.usatoday.com/story/news/nation/2015/05/24/john-nash-dies/27879515/>
• Varian 2006. Intermediate Microeconomics–A Modern Approach WW Northern & Company Inc. New York.
• von Neumann, J. 1937. Über ein ökonomisches Gleichungssystem und eine Verallgemeinerung des Brouwerschen Fixpunktsatzes“ in K. Menger, editor, Ergebnisse eines mathematischen Kolloquiums, 1935–36. [English 1945 trans. as “A Model of General Economic Equilibrium”, RES]