Pythagorean Triples and Other Beasts

I never thought that I would write math stories in English, but I also never thought that I would live in the UK either. I decided to start with small mathematical stories interesting enough to write about them. Today I’ll try to tell you a few interesting facts in number theory.

Let’s have a look at these two circles. Can you guess the difference between them except their differing radii?

From first glance, there is nothing unusual. Just two circles that are laying on the coordinate plane, but in the light of Number Theory, they are completely different as night and day.

I hope almost everyone remembers Pythagorean theorem from school. If we have a right triangle with sides (x, y, z) then:

The triple of such numbers is called a Pythagorean triple. If we continue and divide by z squared we will get:

We can always get this equation from the previous one assuming z is not equal to zero. The second equation doesn’t only allow us to get a Pythagorean triple, but it also defines a point on a circle with a radius equal to one.

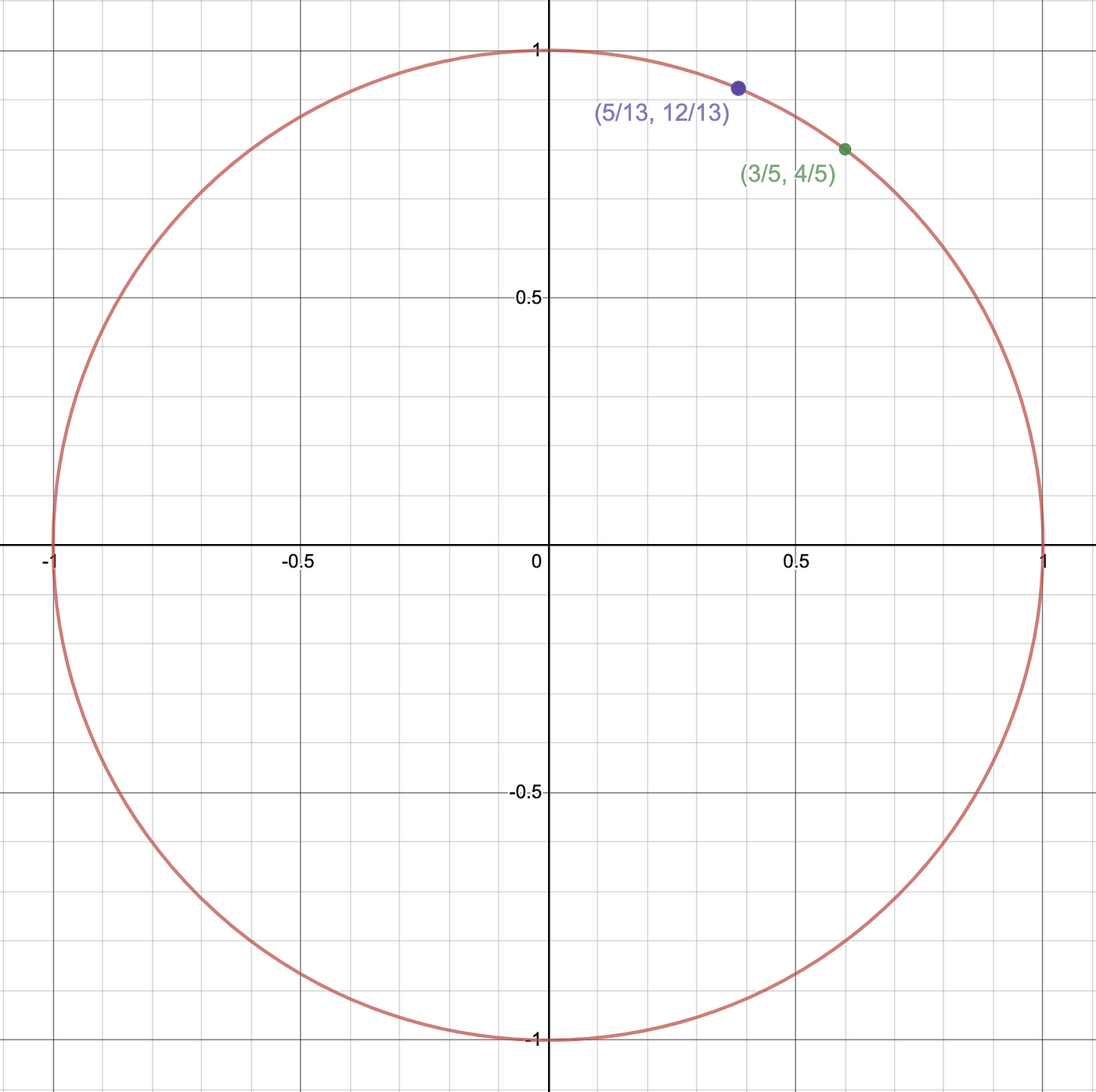
As you can see, we have got a correspondence between all Pythagorean triples and rational points on the circle with a radius equal to one. Can you remember other triples from (3,4,5) and (5,12,13)? Probably, you will need a few minutes to figure out a new one, but it shouldn’t be very hard, because the first fun fact is: there is an infinite amount of such Pythagorean triples. I will try to sketch a proof of it using very simple math.

Let’s draw a line crossing point (-1, 0) and some other point on a circle. This line will be defined using only one parameter t.

This line will cross a circle in two points, and we already know the first one.

The next thing we need to do is to find coordinates of the second point. To do it, let’s try to solve these two equations simultaneously:

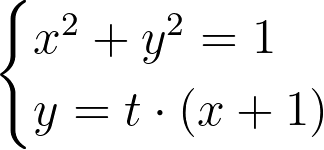
We substitute the line equation into the circle and then simplify it.

As a result, we will get a quadric equation. It should have two roots, and we already know the first one. We can use Vieta’s formula to get the second root.

So, now we have the “x” part of the second point. If we put this value to the line equation, we will get the “y” part.

Now, choosing different rational values of parameter t, we will be getting different points with rational coordinates lying on the circle.

But as we said above, every rational point on the circle would give us a Pythagorean circle. Whoa! Using these simple steps, you can make as many Pythagorean triples as you like. Could you imagine that?

Let’s assume then that for a particular circle, we know at least one rational point lying on it. Using the same reasoning as for the circle with radius one, we will be able to generate infinitely many other rational points lying on the same circle.

So what is the difference between these two circles from the beginning?

If we decide to try our method to the largest circle, we will fail, because our starting point for the line will be irrational. Moreover, all points lying on this circle will be irrational. It is not very difficult to see, but I will leave the proof for the following posts.

Now we should understand the following: there are no circles with a finite number of rational points lying on them! Using this statement, we can split all circles into two sets: with zero amount of rational points and with an infinite amount.

That’s why I like mathematics very much. You can get so cool statements using only school math.

I hope you liked this post.

Stay tuned for the next ones!