Proving the Mean Value Theorem

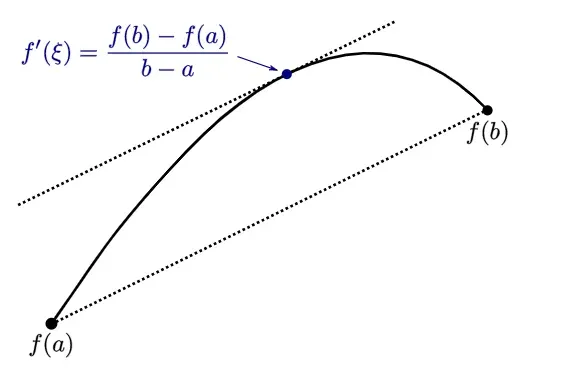
Let’s prove one of the most important theorems in elementary analysis! The only requirement is an elementary understanding of derivatives.

Statement:

Above we see an informal statement.The straight line connecting f(a) with f(b) is equal to the gradient line at one the points between a and b. However, in mathematics it is important to give precise statements as well as visual ones, as images sometimes assume things without you realising it! (e.g. in this example, the curve never dips below the line connecting f(a) with f(b).)

Now the formal statement. Suppose a function is continuous and differentiable on an interval [a,b]. .

Then there exists a number, which we will call c, such that the equation below holds.

Proof

The trick here is to reorient the function.

Consider the function defined below

This new function, which we’ll call g(x), encodes information for how much f(x) has increased relative to the line connecting (a, f(a)) and (b, f(b)). You might have noticed that the (f(b)-f(a)/(b-a) term is the gradient of the connecting line between (a,f(a)) and (b, f(b)).

At x = a, and at x = b, we can use some simple algebra (try it!) to verify that g(a)=g(b).

Now, on [a,b], as g(x) is continuous we can use a result that says g(x) will obtain its minimum value and maximum value on [a,b]. Mathematicians say [a,b] is a ‘compact’ subset of the real numbers.

Then we have two cases.

Case 1. If the maximum and minimum values both occur at the endpoints i.e. at x=a and x=b, then the maximum and minimum values the function can take are the same along all of [a,b] because g(a)=g(b). In slightly less dense terms, if we had a rollercoster, and you knew the start and end points are the same height, and that this point is both the maximum height attained and the minimum height attained, then the height must remain constant for the entire ride. In that case, g(x) is the same everywhere, and some algebra shows that f(x) must then be the straight line connecting (a,f(a)) with (b, f(b)).

Case 2. If either the minimum, or the maximum point occurs in the middle, then we know from calculus, that the derivative must be zero at that point. Let’s call that point c. (You might be able to see where this is heading!). First we work out the derivative:

Then we set the derivative equal to zero at c (N.B. note the switch in notation from df(x)/dx to f’(x) with the ‘ symbol to represent taking the derivative)

Until finally, we conclude, that at this point c, we have

And so, the proof is completed.