About Scientific Theories

By definition, a scientific theory according to Cambridge English dictionary, is a formal statement of the rules on which a subject of study is based or of ideas that are suggested to explain a fact or event or, more generally an opinion or an explanation. Formulating a scientific theory requires an imaginative power to abstract concepts, present them mathematically and be able to prove their functionality in more than one realms. Without being exhaustive, we will try to understand how much effort goes to undertaking such a huge task in this essay, using an example of a new way to look at quantum mechanics.

Perhaps the first question we should be asking is, when do you want to formulate a scientific theory? is it just to attain instant celebrity status? Actually no! in fact theories are the least artefacts that can ever bolster you to fame or riches. Besides, rarely have the proponents of scientific theories derived direct benefits from the theories they propose. This is true however much the theory itself might be successful in explaining what people have always wanted to know, or in clarifying previously formulated theories. Therefore you probably don’t need to come up with a scientific theory. But if you insist, follow along with me.

Who Should Formulate a Scientific Theory?

Scientific theories are community assets geared towards explaining significant phenomena. As such, nobody has monopoly over formulating them. Yes, you heard that right. No one has absolute monopoly over scientific theories. Science is a contest all open to everyone. Access to science (including formulating scientific theories) should therefore be open to any person having the necessary ability and skills to undertake science well enough to meet accepted standards of science, and would not be hampered by financial monopolies¹. However, depending on what the theory is attempting to explain, there is a set of unwritten rules that forms the bare minimum criteria to warrant who can formulate a truly believable theory.

To take an example of General relativity, the proponent Albert Einstein was very particular about falsifiability of a theory — a principle that states a theory must be demonstrated to be false or refutable by observation. A theory must also start by explaining the flaws of the currently accepted scientific norms, and that underscores the need to first have sufficient general understanding of the grounds of the claim. Now, let’s have a fresh look at quantum mechanics.

The Theory: A New Look on Quantum Mechanics

If you have been lucky to get a grasp of the general principle of quantum mechanics, you will have realized that unlike classical mechanics, it describes the wave properties of the objects rather than the objects themselves. For instance, when a stone is thrown, describing the path rather than its speed, kinetic energy, or momentum is likely to be what you want to do. In turn, you describe exactly the same properties, but in the context of the waves generated by its movement. A crucial parameter in most cases is the wave function, described as a variable quantity that mathematically describes the wave characteristics of a particle. It is therefore a mathematical function that estimates the probability that the particle will be found at certain locations along the path of the object — and this marks the beginning of the problem.

The Limits of Quantum Mechanics

Quantum mechanics is difficult for a reason, and if you have ever considered yourself dumb for not being able to understand, don’t worry — you are not alone. American physicist Richard Feynman and Nobel Laureate is often quoted² saying this:

“I think I can safely say that nobody really understands quantum mechanics”

Feynman was arguably the crème de la crème and for that reason it is still safe to accept that he knew what he meant by the above statement. Besides Feynman’s lamentations, quantum mechanics as it is now, is limited. One of the fundamental undoing of quantum mechanics is that we mostly use a probability function to estimate where a particle will be found when a wave function collapses. This means we discretize the path followed by the object, but then we end up focusing on a really small area where the particle actually is — see Fig. 1.

The weird thing about it all is that all paths are possible. That is right. An object can take any conceivable path, including directly opposite to the applied force — you can think of a fluid jetting out of a nozzle in the same way. The quantum mechanics understanding demands that the path back into the nozzle is also possible. Rather, out of pure randomness a fluid particle might just get sucked back into the nozzle.

“…. as long as a particle is in motion, it has a higher probability that it exists in more than one places than it is to exist in exactly one position”

Deriving an Ideal Wave Function

An ideal wave function would be the exact opposite of what we currently have by definition. That is to imply, we need a wave function that quantifies the probability that an object would be found everywhere or in all the existing positions— rather than just one. You will get to understand what this means in the following thought experiment.

Supposing that you were to track a billiard ball Fig. 2, accelerated around a circular path of radius, r. In the strictest quantum mechanics interpretation, the only permanent objects are the positions occupied by the entities we name as particles in motion.

Mathematically, this means that due to uncertainty, the object and the path taken must be discretized or quantized into several small finite numbers. Thus if the quantum mechanical description is to be applied to every day classical objects, such as a ball thrown, we would want to find a function estimating the total number of positions occupied by the particle at the same time. For context, an electron in the first energy level of hydrogen occupies 355 positions (out of the possible 360) at the same time — we should be able to demonstrate this at the end of the exercise.

Theoretically, as you increase the speed of the billiard ball, the object tend to increase the number of (super)positions it occupies along the circumferential path, cₚ = 2π r (at the same time) — this is how quantum superposition builds up. This implies that the path can be quantized into N finite positions such that N = 2π/dθ.

Where dθ is a small fraction cut out of the circular path — in radians. Hypothetically, the number n of position vector, r is a function of the angular velocity, ω. Angular speed ω is in turn a function of path radius r, and linear (tangential) velocity, v, which is in turn a pure function of time, t. You would then want to write the complete chain of equations — from Chain rule as follows:

The full formulation for the rate of change in n with time results in the form in Eq. (2).

The general forms of n for both angular and linear motion respectively can be written as follows:

At the speed of light c , the angular speed becomes ω = c/r, and at this point the particle occupies all the positions along the path. To further avoid triviality, It must be stated upfront that ω ≠ 0, and similarly, v≠0. From first principles of quantum mechanics, we should be able to estimate using this method, the probability, P(r,t) that the particle can be found in one or more of the positions, Nᵢ marked in Fig. 2. The probability would be expressed as follows:

This can also be extended to linear motion, but in a special case in which N does not recur as of the case in angular motion. For linear motion, the expected total path, L is quantized in to N distinct numbers such that N =L/dx. For an event that many outcomes are expected, the shortest path yields a lower number of N, and vice versa, since the division dx would be preferred constant.

Finally, there will be a wave function for each number path and quanta, N, such that the probability function is related to a universal wave function by the following means:

The thesis of this thought experiment is that as the billiard is accelerated towards the speed of light, it starts to exhibit peculiar behaviour of occupying more than one quantum states/positions at a go. The exact probability function would then be given as follows:

Similarly, the universal wave function estimating where the billiard would be found can be expressed as follows:

A closer look at Eq. (7) reveals an astonishing fact that as long as a particle is in motion, it has a higher probability that it exists in more than one places than it is to exist in exactly one position.

Applications of the derived wave function

You might be asking yourself what is the use of all these formulae thrown about, and here comes the explanations. Our aim was to show that objects behave differently in the quantum mechanical context. We gave an example of a billiard ball accelerated in a circular path and said that in the very strictest sense, as long as it is not stationary, it occupies more than one place at the same time. But why aren’t we seeing that? Well, because of our human limitations. Take for example, we linearize Eq. (4) into the following form:

Bohr Atomic Model Calculations

Supposing that we had an electron orbiting the nucleus at the second energy level n=2. We want to estimate the electron’s (linear) speed, v. This method calls for quantization of the travelled path into N finite numbers. Let N = 360. Since n(x,t)=n(r,t) is the only unknown, we immediately deploy the equation to determine n(x,t) followed by the speed as follows:

Where α is a constant value of 1/137.06 — referred to as the fine structure constant. Did we even have to explain why we are using the energy level as shown above? we don’t need to, because such are the mysteries of formulating a theory. Surprising outcomes is the norm. Assuming we now have the number of positions that an electron occupies along that orbit at the same time, we can now proceed and estimate the speed by plugging in n(r,t) in Eq. (8) to get an exact speed of v = 1093624.7384226(8) m/s. You can try the energy level 1 too, and you should end up with the ground-state speed of an electron in an hydrogen atom v = 2187249.47684535 m/s. At the speed of light c, n(r,t)=N making the exponent in Eq. (8) equal to 1.

Solving Special Relativistic Calculations

If you have bothered to ask yourself where problems arise in special relativity, you might have known that they mostly arise from the squared speed ratio v²/c². This is usually a pain in the neck, especially as v tend towards the speed of light in a vacuum. It turns out that with this method you can actually get some relief since now you can write:

Addressing General Relativity Calculations

Curiously, can these models be applied to carry out some predictions concerning planetary movements in space? Well, as it turns out, it is possible to analyze any motion be it in the sub-atomic or in the macro level. In deed you can map the planetary bodies onto a table of precise parameters as shown in Table 1:

Even though we know that electrons do not orbit the nucleus like planets do, the implication of above model is that we can spot a planetary object,
measure its speed, and if we asked were it that we are residing in an atom, what would be the equivalent energy level of our planet — the answer would come up from this analysis. The method also goes to show that there is indeed a connection between general relativity and quantum mechanics, albeit a hidden one.

Explaining Atomic Energy Levels

The notion of energy levels was proposed in 1913 by the Danish physicist Niels Bohr in his atomic model theory. The modern quantum mechanical theory explains these energy levels in terms of the equation developed by Erwin Schrödinger and Werner Heisenberg in 1926. While these nuclei shell numbers (denoted n) have been used for a long time without necessarily knowing how else they arise — a part from Rydberg’s formula, this theoretical method has proven handy in further explaining their origin. With the j estimated from Eq. (10), it is now apparent that n is derived from the fine structure constant as follows:

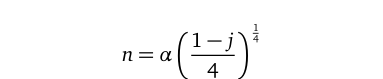
For every value of n for instance in an hydrogen atom, there would be corresponding values of n(r,t) being the number of positions occupied at the same time, and probability P(r,t) such that given N = 360 as an example:

Comparison to Particle in a Box Model

Particle in a box model is a classical example mostly used to introduce students to quantum mechanics. As earlier stated, all calculations in quantum mechanics tries to estimate quantifies the probability that a particle would be found in a certain location at a certain time interval. For instance, the wave function for a particle in the n to the power of nth energy level state in an infinite square well with walls x=a and x=b is given by the following function:

In this particular case however, we expect that the probability, p(x,t) that the electron will be confined to one position is given by taking the integral of the square of the wave function and subtracting it from unity to obtain the conjugate as follows:

It therefore implies that if the speed is known as a function of the energy
level, N is found by discretizing the position the travelled length into definite positions and the probability estimated.

Conclusion

A general overview has been given on when a scientific theory is needed, and who can formulate one. We then ended up undertaking an example through abstraction. The thought experiment resulted in a primitive, yet functional form of a wave function, which is consistent with Bohr model at the lowest level, and also to some astronomical applications we know. The ultimate aim of these developments is to answer the questions that many people ask, including some prominent physicists — is our universe quantized? A thorough investigation of the views in this article would not only answer such questions, but may also provide a window for the grand unification of quantum mechanics to general relativity — a quest currently pursued by many physicists.

If science was a religion, scientific journals would be the bible, mathematics would be the language, and imaginations would be the prayers

References

¹ Radder, H. (2010). The commodification of academic research. The commodification of academic research: Science and the modern university, 1–23.

² https://www.nytimes.com/2019/09/07/opinion/sunday/quantum-physics.html