How to Compute the General Relativistic Correction to Time

General relativity is one of the most technically difficult physics theories, as it requires uncommon knowledge and mathematical skills. However, it is possible to calculate the relativistic effects induced by the curvature of space-time with good precision, and even to derive its formula, with a few simple arguments.

We know from special relativity that time flows more slowly in a moving system than in a stationary one. Given t as the time elapsed aboard the moving system, the time elapsed for the stationary one is 𝛾t, with 𝛾>1. In other words, time for those moving flows more slowly. A striking demonstration of this phenomenon is the decay of muons. These particles have an average lifetime, if produced in the laboratory, of about 2 𝜇s: after this time, about 1/3 of the original particles no longer exist. They have transformed (decayed) into electrons and neutrinos. Muons, however, are naturally produced by cosmic rays at an altitude of about ℓ=10 km. If they travelled at the speed of light c=3×10⁸ m/s, they could travel no more than 600 m in 2 𝜇s. If they travel 10 km it means that their life must last (for us, who see them moving) a little more than 30 𝜇s (ℓ/c): that is, they must have a 𝛾 of about 15.

According to general relativity, a gravitational field is (locally) indistinguishable from an accelerated system. In practice, being in an intense gravitational field is like being in a strongly accelerated system, while in a weak field the perceived acceleration is weak. If, in a moving system, the flow of time is altered, the same must happen in an accelerated system and, therefore, in a gravitational field. Of course, said t the time elapsed on board the system immersed in the gravitational field (equivalent to a system in motion), the time seen by an observer outside this field will be 𝛾t, where 𝛾, this time, is still a dimensionless number (because 𝛾tmust continue to have the physical dimensions of a time) that, however, does not depend on the speed of the system, but on the intensity of the gravitational field. If the latter is zero, 𝛾=1.

We can then write that 𝛾=1+𝛿, where 𝛿 must depend on a scalar quantity that determines the intensity of the field. The gravitational potential V=GM/r is a good candidate: G is the Newton’s constant, M the mass of the field source and rthe distance from it. This is a scalar quantity that determines how intense the gravitational field is (the field intensity is its derivative): the greater the mass generating the gravity, the greater 𝛿. The closer we are to the source, the more curved space-time is, i.e. gravity is intense, and consequently 𝛿 increases as we get closer to the source. However, we cannot write that 𝛿=GM/r, because, due to the above, 𝛿 must be dimensionless, while GM/r is not.

It is easy to see that the dimensions of the gravitational potential are those of a velocity squared. A number proportional to the potential, yet dimensionless, can therefore be constructed by dividing the potential by a velocity squared. But which one? Obviously that of light, which is a universal constant. Thus

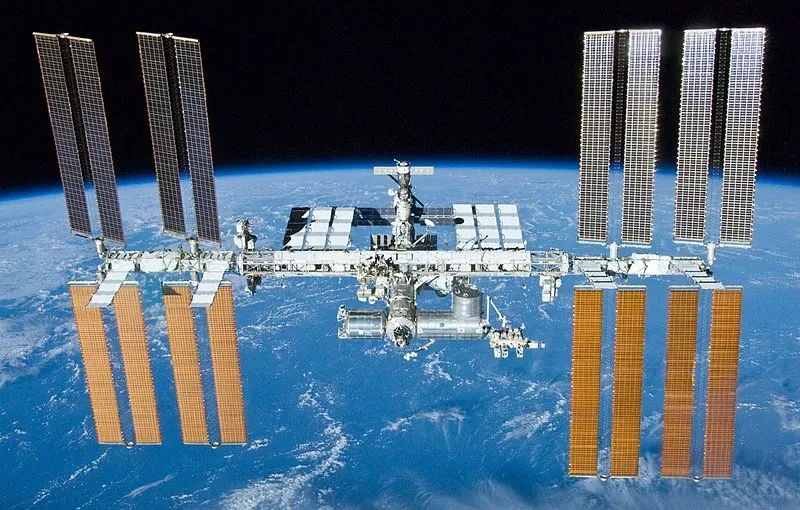
is the correction sought. A clock on Earth runs slower than one in deep space because, on Earth, one second lasts a factor of GM/(c²R) longer, where R is the radius of our planet. The consequence is that a clock on the ISS, which orbits at an altitude h=400 km, runs faster than one on Earth, because the relativistic correction is smaller: GM/(c²(R+h)).

It turns out that the exact calculations, which can be made from Einstein’s equations, lead to a result that is exactly the same. The only difference, indeed, could be a numerical factor in front of the potential, but this factor happens to be exactly 1. Cool! Isn’t it?

GPS systems could not function if we did not take this phenomenon into account. In fact, the running of the onboard clocks is artificially altered to compensate for the combined effect of special relativity (which slows down the clocks) and general relativity (which makes them faster)