When I was a small child I got lost at a store with lots of aisles. I remember being very scared that my mom would never find me. I walked from aisle to aisle on the verge of tears hoping to run into her. Eventually, to my great fortune, I did.

However, it occurs to me that had my mom and I started looking for each other at opposite ends of the store, our paths inside the store been constantly reflected over the center of the store, or had it been a store with an odd number of evenly-spaced aisles, I would have still been wandering those aisles, just barely missing my mom for the rest of time.

As eerie as this thought experiment may be, It has some interesting mathematical properties that are fun to consider.

A Simple Model

Imagine two points on a plane that can travel anywhere on the plane at the same speed. Between them lies an obstacle. Perhaps the obstacle is just a static point.

The motion of the red points is restricted by the condition that at any given moment, the red points are reflections of each other over the blue obstacle point.

If the first red point approaches the obstacle, so does the second one. If the first red point moves away from it, then so does the second one.

We’ll say that the red points can ‘see’ each other if one can draw a line that goes through both red points without being blocked by the obstacle point.

The question is: can the two red points find a position where they can ‘see’ each other?

The answer is predictably no: the points will never be able to ‘see’ each other. The reflected motion condition implies that no matter where the two red points move, the line going through them will also go through the obstacle point. Therefore in every possible configuration, the obstacle will block the red points from directly ‘seeing’ each other.

This principle can be extended to various obstacles and to situations where this reflected motion comes about naturally. Here is a real-world thought experiment with some surprising ramifications:

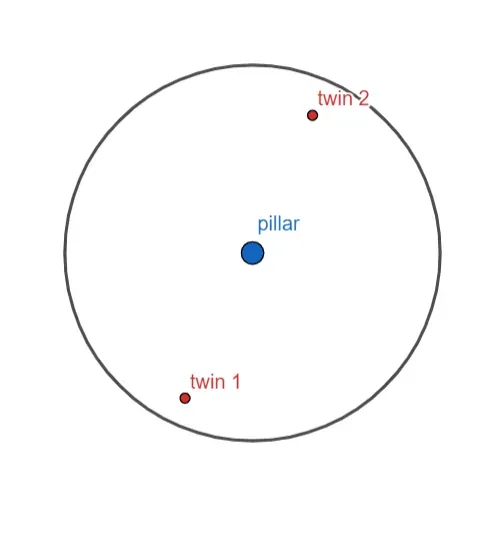
Lost Twins Experiment

Imagine two genetically identical twins that have been conditioned in an absolutely identical way. Ask them to close their eyes and lead them to stand on opposite ends of a perfectly symmetrical rotunda with a pillar in the center just big enough to obstruct the twins’ view of each other at every distance.

For the sake of this example, the twins cannot communicate in any way. They cannot speak to the other twin or hear the other twin’s footsteps. As the twins open their eyes and are faced with the same exact angular view of the rotunda, they will begin to move around trying to find their twin. But because they are the same in every conceivable way, their motion around the rotunda will be identical, and therefore mutually-reflected over the center. If either of the twins decides to speed up or abruptly run in the reverse direction from the one they appeared to be going in the hopes of ‘tricking the system’, as long as the twins are absolutely identical in genetics and conditioning, the other twin will decide to do the same thing. This locks the twins in an endless search for one another in a room that doesn’t have to exceed a few meters in area.

In general, the room does not need to be circular and the obstacle does not need to be a pillar. The two conditions that must be satisfied for this experiment to work are:

1. Whatever obstacle you choose must cover the center-point of the room and obstruct the twins’ view of each other.
2. The twins must begin at opposite ends of the room with respect to the obstacle point as mentioned, and their fields of vision must be indistinguishable. Otherwise, at least one of their conditions is not truly identical.

Getting back to the topic of stores and aisles, a store with an even number of evenly-spaced aisles won’t have a center aisle which allows the twins to find each other since the center-point isn’t covered:

A store with an odd number of aisles will likely have a middle aisle, covering the center-point, and could satisfy the above two conditions, meaning the twins will never find each other:

While this situation might seem unlikely and impractical as an experiment, if done correctly, it would allow us to test if human beings are the ‘sums of our parts’, namely our genes and conditions as the determinists claim, or whether there is some third, unpredictable component to our nature that will eventually cause the twins’ motions to diverge.