A conservation law says that some property of an isolated system does not change as the system evolves. As a consequence of Noether’s theorem, which will be the central topic of this article, conservation laws “are associated with symmetries in the underlying physics” (see this link). In other words, symmetries and conservation laws are deeply interconnected.

Quoting the great theoretical physicist and Nobelist Richard Feynman in his physics textbook “The Feynman Lectures on Physics”:

“Why should we be concerned with symmetry? In the first place, symmetry is fascinating to the human mind, and everyone likes objects or patterns that are in some way symmetrical. ”

— Richard Feynman

An example of an object containing several types of symmetries is the one below. This object contains three types of symmetry, namely, reflectional symmetry, rotational symmetry, and self-similarity.

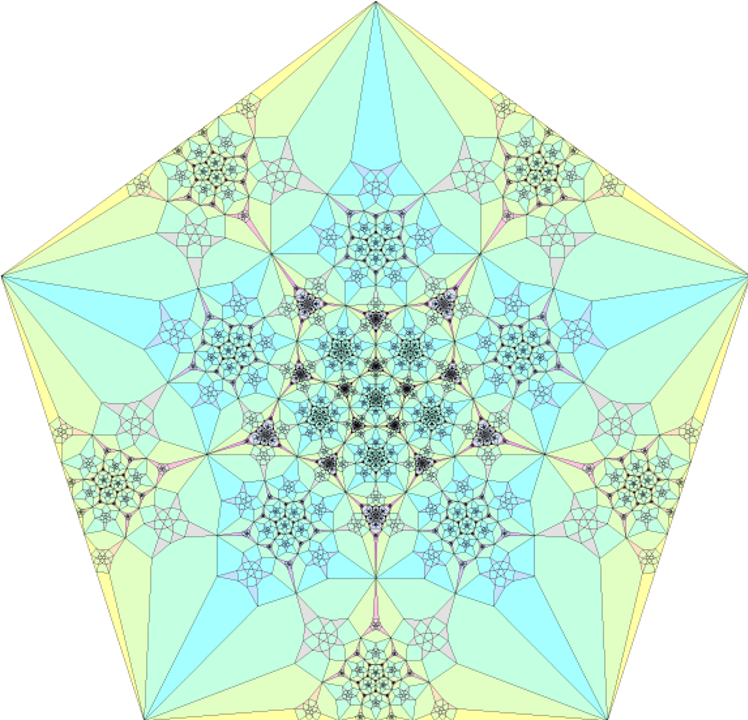
However, we will consider here symmetries in the laws of nature themselves (not just in objects), the laws that govern the behavior of physical systems of the universe. Quoting Feynman again, these are much more “remarkable symmetries.”

Two of the best definitions of these types of symmetry transformations are given by the American theoretical physicist and Nobel laureate Steven Weinberg and by the Chinese-American physicist and writer Anthony Zee in their twoquantum field theory books.

Weinberg defines a symmetry transformation as follows:

“A symmetry transformation is a change in our point of view that does not change the results of possible experiments.”

— Steven Weinberg

Zee gives the following definition:

When a law of physics does not change upon some transformation, that law is said to exhibit a symmetry.

– Anthony Zee

Typical examples include the conservation of energy, linear momentum, and angular momentum. These are associated with the following three spacetime transformations: translations in space, translations in time, and rotations.

The conservation of momentum and energy can be observed using a device called Newton’s cradle, shown below.

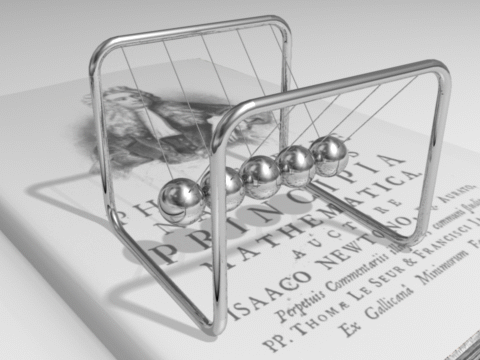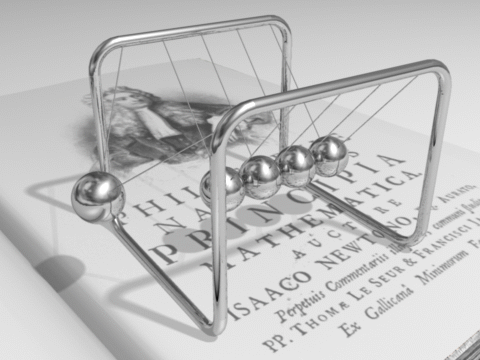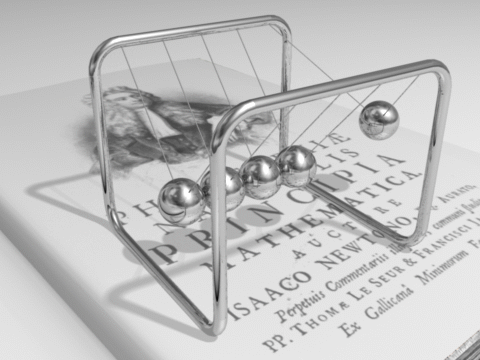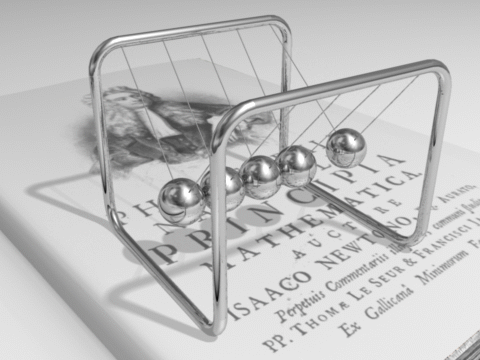
The transformations above are related to symmetries of spacetime. However, fields can also transform inside another (mathematical) space referred to as “internal space.”

As we shall see in more detail, (local) conservation laws usually are represented mathematically as continuity equations. The latter are partial differential equations (PDEs), and give a relation between “the quantity and the “transport” of that quantity.” The former is called the conserved quantity and the latter, the current density. More precisely, continuity equations state that the amount of the conserved quantities “can only vary by the amount of the quantity which flows in or out of the volume containing the system.” As previously mentioned, one of the most important results in all physics, called Noether’s theorem(proved by the renowned mathematician Emmy Noether), states that there is a conserved current corresponding to each symmetry in the underlying physics.

In this article, I will focus on Lagrangian field theory, which is the field-theory correspondent to the usual Lagrangian mechanics (which treats particles).

Let us state this in mathematical form. For that, we first need to understand two important concepts:

• What are Lagrangians and Lagrangian Densities
• What is the Least Action Principle

Lagrangian Mechanics and the Least Action Principle

Lagrangian mechanics was introduced by the mathematician and astronomer Joseph-Louis Lagrange. Though it produces the same results for any physical problem compared to the Newtonian formalism, it has several advantages. For example (see Tong):

• It is exquisite, particularly if compared with Newton’s approach.
• It is much more powerful, allowing physicists to solve challenging problems straightforwardly.
• It is an entirely new way of viewing classical dynamics providing a framework that can extendable to all laws of physics.
• It demonstrates the connections between classical and quantum mechanics.

Lagrangian Mechanics in Classical Field Theory

As it turns out, the equations of motion (the equations that describe how a physical system behaves as a function of time) of all fundamental laws of physics currently known can be obtained from the field-theoretic version of Lagrangian mechanics called Lagrangian field theory. To get the equations of motion of a system, we need first to understand the “least action principle,” which generates such equations.

Consider a system of many scalar fields:

where x = (t, x, y ,z). In Lagrangian field theory, the equations of motion are obtained using the principle of least action mentioned above. For some field ϕₐ it reads:

Let me explain the meaning of the terms in the equation. The curvy L inside the parenthesis is the Lagrangian density, a function that depends on the fields in Eq. 1, their corresponding space and time derivatives, and the coordinates t, x¹, x², and x³. The brackets “{ }” represent n independent variables of the system (including the time variable), the greek indexes μ = 0, 1, 2, 3. Applying the principle of least action to S given above we obtain the equation of motion (or Euler–Lagrange equations) for the field ϕₐ:

Noether’s theorem

This powerful theorem was proven by the relatively unknown (at least for non-specialists) mathematician Emmy Noether in 1915 and published three years later.

Who was Emmy Noether?

The German mathematician and physicist Emmy Noether was one of the greatest unsung scientific heroines of all time. Though she experienced prejudice throughout her brilliant career, her colleagues recognized her almost unparalleled genius.

It isn’t easy to exaggerate the significance of her contributions both to abstract algebra and theoretical physics. Noether’s theorem is a cornerstone of modern physics. Furthermore, her command of modern algebra led the mathematician Irving Kaplansky to state that “It surely is not much of an exaggeration to call her the mother of modern algebra.”

In a 1935 letter to the New York Times, Albert Einstein wrote:

“In the judgment of the most competent living mathematicians, Fräulein Noether was the most significant creative mathematical genius thus far produced since the higher education of women began. In the realm of algebra, in which the most gifted mathematicians have been busy for centuries, she discovered methods which have proved of enormous importance in the development of the present-day generation of younger mathematicians.”

— Albert Einstein

Nevertheless, even after being hired by the University of Göttingen, she struggled to find a paid position because of her gender. Even after the University started paying her, she never became a full professor. The mathematician and physicist Hermann Weyl, one of the most prominent scientists of the time, said,

“I was ashamed to occupy such a preferred position beside her, whom I knew to be my superior as a mathematician in many respects.”

— Hermann Weyl

In her obituary, the mathematician Bartel Leendert van der Waerden wrote that her creativity was “absolutely beyond comparison.” She was also described as one of the greatest mathematicians of her time by exponents such as the Soviet mathematician Pavel Alexandrov and the great French mathematician Jean Dieudonné.

The Mathematics of Noether’s Theorem

Let us start by considering a continuous symmetry, and an infinitesimal variation of the fields δϕₐ. The Lagrangian density does not change:

Now, to proceed, we need to use the Euler–Lagrange equations. The two equations together give us:

We then define the object inside the parenthesis as a 4-current J:

The previous equation states that J is conserved:

The Conservation of Energy and Momentum

In this section, we will investigate two types of transformations:

• A translation is space is a transformation that moves every point of a figure or a space by the same distance in a given direction (from Wiki). In this case a continuous translational symmetry is the invariance of a physical system of equations under any translation (see this link).

• A translation in time is a transformation that moves the times of events by a constant interval. Time translation symmetry is the assumption that such a shift does not change the laws of physics.

We can write both transformations as:

where x⁰ =t, x¹=x, x²=y, and x³=z and a is a small arbitrary parameter characterizing a displacement in spacetime. Now consider a scalar field ϕ(x). If we vary it by a small perturbation

and use the Euler–Lagrange equations we find after a few lines of algebra the variation of the corresponding Lagrangian, which depends on the coordinates tand x:

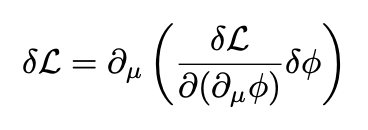
Using Eq. 9 we obtain:

Now, we can write the variation of the Lagrangian density as:

Comparing with Eq. 11 and recalling that a is arbitrary we obtain:

The expression inside the parenthesis is called energy-momentum tensor and Eq. 13 can be written as:

The 00-component of T is the energy density of the system and the i0-components of T (for i=1,2,3) are the components of the momentum density of the field. Integrating over all space we obtain the energy and the three momentum components. Eq. 14, therefore, expresses the conservation of energy and momentum when the spacetime translation Eq. 8 is performed without changing the Lagrangian density (in fact, since only the action S needs to be invariant, as shown in Eq. 2, the Lagrangian density could contain an extra total divergence but I will omit this issue here for brevity).

As mentioned before, fields can also transform inside “internal spaces.” An example of a continuous symmetric Lagrangian, where the symmetry transformations are internal (they are rotations in ϕ space) is:

Conserved Currents

Let us consider again a transformation of a scalar field. It implies that:

Suppose now that the Lagrangian does not change. Proceeding exactly as in the last section we find the following result:

where J is called current density 4-vector. The 0-component is the charge density and the i=1,2 and 3 components are the 3-current density (charge per unit area per unit time). Hence, we finally arrived at the main result of Noether’s theorem, which I shall repeat here for convenience:

Every continuous symmetry of the Lagrangian of a physical system has a corresponding conservation law. In other words, when the Lagrangian is symmetric there is a conserved charge associated with each conserved current.

The most obvious example (cited above) is the conservation of charge:

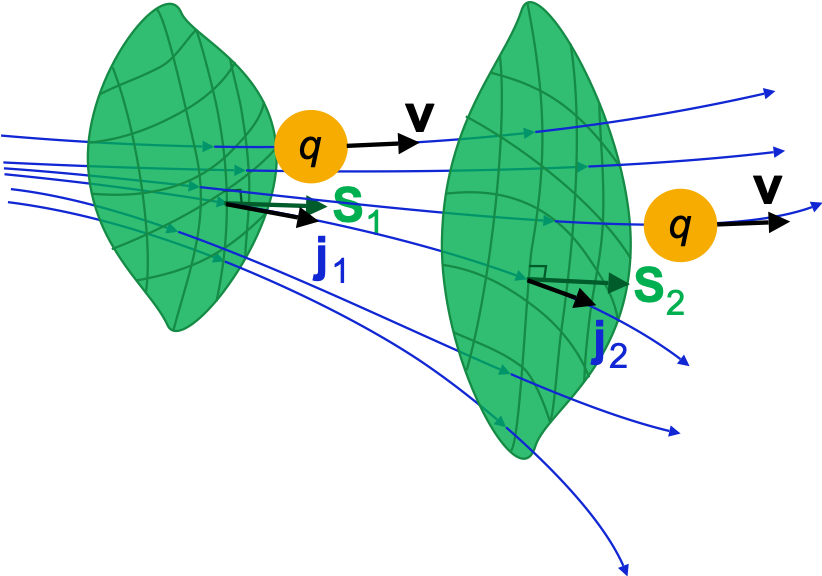
Noether’s theorem can be used in a multitude of different systems including the electromagnetic field, gauge theories in general, and many other systems. This article was about the classical version of the Noether theorem but it can be easily extended to quantum mechanics and quantum field theory.

Thanks for reading and see you soon! As always, constructive criticism and feedback are always welcome!

My Linkedin, personal website www.marcotavora.me, and Github have some other interesting content about physics and other topics such as mathematics, machine learning, deep learning, finance, and much more! Check them out!