It’s one of the best-known thought experiments in physics. A cat is placed in a sealed box with a vial of lethal cyanide gas which will be released if a radioactive atom is detected to have decayed. Since the decay of the atom is probabilistic, quantum mechanics tells us that we can describe the cat as a quantum superposition of an “alive” and a “dead” state until the box is opened and the state is observed.

Erwin Schrödinger proposed his eponymous cat experiment in 1935 not as a proponent of the quantum theory of the time, but as an opponent. The idea that a system so classical as a cat could exist as a quantum superposition was intended as the absurdity in what is almost a dis-proof by contradiction of the Copenhagen interpretation of quantum mechanics.

So, what is this interpretation which Schrödinger opposed? Developed by Danish (hence Copenhagen) physicist Niels Bohr soon after the birth of quantum theory along with Werner Heisenberg, the Copenhagen interpretation is often seen as the “textbook” interpretation of quantum mechanics.

Its main tenet is the existence of the wavefunction: a description of the system which contains all the information which could be known. The wavefunction exists as a superposition of possible “results” of a measurement, known as eigenstates, each with a certain associated probability.

Crucially, under the Copenhagen interpretation, when we observe or measure a quantum system, the wavefunction collapses into one of its component eigenstates. Only then is the result of a measurement well-defined.

For example, let’s imagine I have a quantum coin, i.e. some quantum system which when measured returns one value which we call “heads” or another which we call “tails” with equal probability. In quantum terms, we say that before it is “flipped”, the quantum coin can be described by a wavefunction which is an equal superposition of the “heads” eigenstate and the “tails” eigenstate. When we “flip” the quantum coin by observing the system, the wavefunction collapses into either the “heads” eigenstate or the “tails” eigenstate, giving us the result of the coin “flip”.

So, imagine we perform the Schrödinger’s cat experiment, and that when we open the box, our cat has shuffled off its mortal coil. The big question is this: when did the cat die? Under the Copenhagen interpretation, the state of the radioactive atom is only determined when the system is observed upon opening the box, and since the fate of the cat is uniquely dependant on the state of the atom, the cat only dies when the box is opened. Until this point, the atom exists as a quantum superposition of a decayed state and an undecayed state, so the cat exists as a superposition of being alive and dead.

This doesn’t make sense, however, since a cat is far too big an object to be able to exist in a quantum superposition. If a cat can’t exist in a superposition, then the subject of our experiment must have died before the box is opened and the system is observed. This is our contradiction: the Copenhagen interpretation requires an object which cannot exist as a superposition to exist as one!

The general form of this paradox is known as the Measurement Problem. How exactly do the probabilities of a quantum mechanical system return a well-defined outcome when we perform a measurement? The Copenhagen interpretation provides one answer to the problem, as we have just seen, but there are other interpretations of quantum mechanics which provide their own resolutions.

A Deterministic Solution?

Among the oldest alternatives to the Copenhagen interpretation are the hidden variable interpretations, whose supporters included none other than Einstein himself. These are based on the idea that the wavefunction does not contain all the information about the quantum system. This extra information — the hidden variables — is inaccessible to us through measurement, and the apparent probabilistic nature of quantum mechanics is as a result of our lack of knowledge of these variables.

In a similar way to how we could predict the result of a coin flip if we knew the exact initial conditions of the toss, a hidden variable theory would claim that knowledge of the hidden variables of a system would allow us to accurately predict, for example, the result of flipping our quantum coin.

One such hidden variable interpretation was proposed by one of the fathers of quantum theory, Louis de Broglie, in the 1920s and expanded in the 1950s by David Bohm. It treats the wavefunction as a pilot wave carrying quantum particles with it through space, just as an ocean wave carries a beach ball. The hidden variable here is the particle position: there exists a function which describes the position of the quantum particle under the influence of the wavefunction. If we knew this function, along with the initial position of the particle, we could predict where it would be at any time, just as if it were a classical particle.

Importantly, there is no wavefunction collapse involved in the measurement of a particle under the de Broglie-Bohm interpretation. Here, unlike under the Copenhagen interpretation where a system has its own, independent wavefunction which governs its behaviour, there is a universal wavefunction which determines the trajectories of particles. The universal wavefunction never collapses. The probabilistic behaviour we previously assigned to wavefunction collapse is now a consequence of our lack of knowledge of the hidden variables of the system.

This idea allows us to do away with the measurement problem in the Schrödinger’s cat experiment because the decay of the radioactive atom is now fundamentally deterministic. Each of the particles in the nucleus follow a well-defined trajectory under the influence of the wavefunction of the system. If we knew the information required for such a calculation, we could predict with 100% accuracy whether the atom will have decayed, and thus whether the cat will have died. There is no longer any need for the quantum superposition of “alive” and “dead” states of the cat which plagues the Copenhagen interpretation resolution to the problem.

The Infinite Multiverse

While de Broglie-Bohm solves the measurement problem by allowing hidden variables, other alternative interpretations maintain the wavefunction as the sole repository of information about the system. One of these is the Many Worlds interpretation proposed by Hugh Everett in 1957 and later popularised by Bryce DeWitt.

Similarly to de Broglie-Bohm, Many Worlds describes the universe in terms of a universal wavefunction. This universal wavefunction also never collapses. Instead, when a measurement is performed on a quantum system, the wavefunction becomes a superposition of non-interacting timelines, each of which contains one result of the measurement. Since the wavefunction describes the universe at large, this can be thought of as the universe itself splitting when a measurement of a quantum system is made.

The separation of the timelines is described by a mechanism known as quantum decoherence. This regards a measurement apparatus or an observer as a quantum system in itself — something which is impossible under the Copenhagen interpretation — and describes the entanglement of the observed system and the apparatus upon measurement.

This entanglement introduces a dependence of the state of the observer on the result of measuring the system, meaning the universe in which a particular result is found is incompatible with the universes which contain the other possible results of the measurement. Thus, the timelines are said to be decoherent: the wavefunctions describing the universes of each timeline are unable to interfere, resulting in the classical certainty we are used to after measurement without the need for the wavefunction collapse of the Copenhagen interpretation.

For example, the two possible measurement results of our quantum coin are “heads” and “tails”. Before we make a measurement, the universal wavefunction is a superposition of a timeline where the quantum coin is in the “heads” state and one where the coin is in the “tails” state when I measure it, and these timelines can happily interfere with each other, resulting in the quantum behaviour of the coin before measurement.

But, when I make the measurement, my apparatus becomes entangled with the quantum coin in a way which depends on the result. The universal wavefunction which describes the timeline in which I measure “heads” is fundamentally different to that which describes the timeline in which I measure “tails”. The quantum coin is no longer in a superposition. Rather, we have two decoherent timelines, each described by their own universal wavefunction that cannot interfere with that of the other timeline. We have, in effect, two parallel universes, the same except for this one result.

Since quantum interactions occur a truly unimaginable number of times per second throughout the universe, the Many Worlds interpretation concludes that there must therefore exist perhaps infinitely many universes parallel to our own, with each marginally different to the next.

We can use this to solve our Schrödinger’s cat problem, since under Many Worlds, the universe splits into decoherent timelines when the box is opened, one containing a living cat and one a dead cat. Since the timelines cannot interfere, an observer in each timeline only ever sees the cat unambiguously alive or dead.

Does any of it matter?

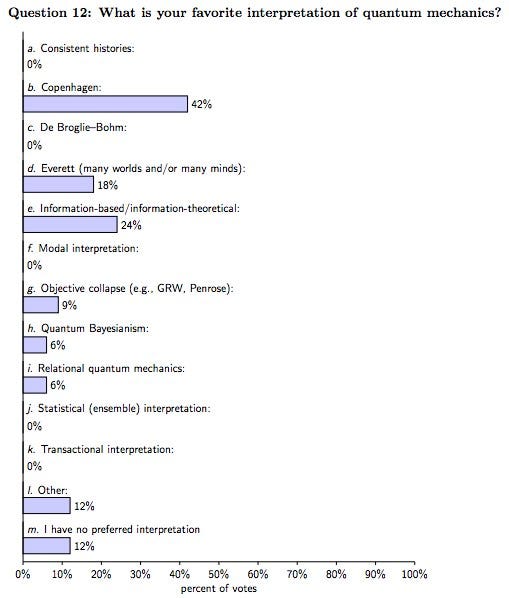
So, one might ask, which of these is right? There is no simple answer, which is why such ideas are, for the large part, said to be interpretations instead of theories of quantum mechanics. All have their advantages, and all have their weaknesses, as do the rest of the menagerie of interpretations out there.

We have already seen that Copenhagen fails to adequately explain wavefunction collapse in its original form, but some would argue that this problem is solved with quantum decoherence as a mechanism to collapse the wavefunction.

Others support the return to determinism of de Broglie-Bohm, with results consistent with quantum experiments without the need for wavefunction collapse at all. But here Occam’s razor steps in. Why should Copenhagen be inferior if de Broglie-Bohm gives the same quantifiable predictions but with the extra assumption of hidden variables which, by their very nature, cannot be detected?

Possibly more intellectually challenging is Many Worlds. While it provides a description of the quantum world which is both deterministic without the need for hidden variables and more rigorous than Copenhagen regarding the measurement problem, the implication of an infinite tree of parallel universes is troubling and potentially unfalsifiable, given that an observer can only ever consciously experience one timeline. This, combined with the interpretation’s current lack of explanation for why probabilities behave as they do in quantum measurements, is a possible reason to doubt the validity of Many Worlds.

Perhaps a larger question is why does anyone care? Quantum mechanics is one of the best tested theories ever devised, and all interpretations are attempts to recover the results of experimentation in the theory. Many, notably Feynman and Dirac, would say “shut up and calculate!”: the reason why quantum mechanics behaves as it does is irrelevant so long as we can use its results.

I would disagree. While it may not provide the breakthrough which gives us quantum computing or a high-temperature superconductor, interpreting quantum mechanics provides a peek behind the curtain of our universe. I would argue that the purpose of science is to not just provide routes to new technologies, but to better understand the world around us whether it is “useful” or not. To successfully interpret quantum mechanics might well be to understand the universe on its most fundamental level, which makes it, in my opinion, one of the most important goals in physics today.

And while Schrödinger’s Cat is, thankfully, just a thought experiment, it provides a particularly useful lens to examine this very fundamental nature of the quantum realm. Though we may not yet have a universally accepted interpretation of quantum mechanics, the framework we’ll use to dissect it once it comes along is already well established.