Albert Einstein has been one of the most celebrated scientists of all time. It is funny, that his ideas are also, among the most difficult to understand, and many people do not quite know what he did, and how he did all those things. It is the mystery of not knowing how the mind of a scientist works, that creates an aura of greatness. It takes years of study, everlasting passion and a great deal of luck, to come up with something worth celebrating.

One thing that is common to all great scientific breakthroughs is that — most new ideas are almost always driven by old ideas that have been working well for a long time, and somehow begin to fail under special conditions. A scientific progress is always made by small incremental changes. The only exception is — when we make an accidental scientific discovery, which usually opens up a Pandora’s box, often leading to significant advancements in a very short amount of time.

The purpose of this article is to make an attempt on demystifying the process of making incremental changes, which may lead to a significant breakthrough. We are going to study in detail, an already established law of nature, and try to improve it.

An Established Law of Nature

Galileo di Vincenzo Bonaulti de Galilei (15 February 1564–8 January 1642) was an Italian astronomer, physicist and engineer. He is widely recognised as the “father of modern science”. Most importantly, he is often credited with the development of an idea called “The Scientific Method”, which is the basis of allmodern sciences. He studied the motion of falling objects, and discovered the law that could explain most of the observations (within the limits of his experiments).

We are going talk about the mathematical form of this law, and try to come up with an improved version, without invoking too many new ideas. The intention is to construct a systematic method where we can improve existing laws, with just a little bit more information than we already have. This may not be possible every time, but there are cases, where this could be very useful.

Galileo’s Law of Falling Objects

The law of falling objects was discovered by Galileo, much before Issac Newton came up with his theory of gravitation. As far as Galileo did experiments, the observations matched the predictions made by his theory. But, just like any other theory, there could still be an experiment, designed specifically for the purpose of testing the limits of the theory, where it breaks down. Actually, this idea of testing a theory to its limits is a relatively modern concept, which in Galileo’s time was just starting to be developed.

It turns out (as we will see), that his law doesn’t work as well for objects falling from heights much greater than a certain range.

Today, the most common (and better) explanation of the behaviour of falling objects requires the understanding of Newton’s law, which is usually presented as an ad hoc rule that he, just like Galileo, found to be working well for a lot of cases. It is not unimaginable that Newton was significantly influenced by Galileo’s discoveries, which eventually led him to a better theory of falling objects.

Here we are interested in knowing if its was possible for someone who lived before Newton, and knew about the limitations of Galileo’s law, to come up with a significant improvement.

Let us begin by stating Galileo’s law of falling objects -

when starting from rest, a falling object acquires, equal increments of speed, during equal time-intervals.

It means that if we drop an object from a certain height, it will fall down twice as fast after two seconds, as it was falling after one second. It will fall down thrice as fast after three seconds, and so on. In other words, a falling object continuously (uniformly) picks up speed until it hits the ground. We call such a motion as accelerated motion.

The mathematical form of this law is really simple, and is written as,

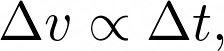
were Δv is the change in speed, and Δt is the corresponding change in time.

We can fix the proportionality constant, with a number that does not depend on the properties of the falling object in any way. It should be some sort of a universal number that works for all objects falling towards earth. We write this as,

Now, one way (sometimes, the only way) to obtain the numerical value of a is by using the data from a set of sufficiently large number of experiments involving falling objects. Here, we need to measure the speed and the duration of time. In practice, measuring the duration of time interval through which the object falls is quite simple — by using a clock. But, measuring the change in speeds is not quite as simple, because getting hold of a speedometer for this particular setup is relatively harder, and certainly a lot harder in Galileo’s time.

Luckily, we have an alternative. We know from everyday experience that the distance travelled by an object moving with constant speed for a certain amount of time is simply the product of its speed and time. We could use this result, to convert the changes in speed into changes in distances which are practically much simpler to calculate. The only problem is — Galileo’s law clearly states that the speed of a falling object is not constant — it increases over time. So unfortunately, we cannot make this replacement straightaway, but we can imagine that even though the distance is not exactly equal to the product of speed and time, it surely increases much faster with increasing speed. This means that instead of an equality, we could try a proportionality like the following,

where h is the height through which an object falls in time Δt , during which the speed changes by an amount Δv. We may believe that this equation looks reasonable, but there is a possibility that it might not be completely correct, because there are so many other equally valid equations, that could at least partially explain our physical phenomenon. But, the best we can do right now is to try mathematically simpler guesses, for as long as we do not find experimental evidence that says otherwise.

Now, we use the formula of Δv obtained earlier, to obtain the height through which the object moves during an interval of Δt, as

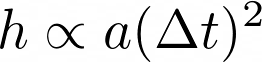
Again, we have a proportionality to fix, and we use another constant b , to get

Here we have a product of two unknown constants, and we cannot determine their values simultaneously using a single equation like we have here. All we can do is to obtain directly the product, which is fine for our purposes. Historically, this is exactly what Galileo did — He dropped two objects (supposedly from “The Leaning Tower of Pisa”, but it is more likely that he performed a much simpler experiment with two objects falling along an inclined plane of a given height, or something similar), and recorded the time they took for travelling through different heights, and found that this equation worked well for all cases. From the experimental data, the numerical value of ab turns out to be approximately 5.

It is interesting to know that there is another way of obtaining, just the value of bwithout even doing any experiment. It involves the use of calculus, which Newton is going to invent a few decades later! (Unfortunately, no such clever mathematics can lead us to the numerical value of a.)

Now, every physically measured quantity must have units (like meter, kilogram, second), and we need to find the units of a and b. We do that by writing the original form of Galileo’s law as

The standard units of measuring speed and time are meter per second (m/s), and second (s) respectively. So, we can write this equation as

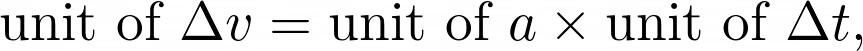
and from this, the unit of a is simply obtained as

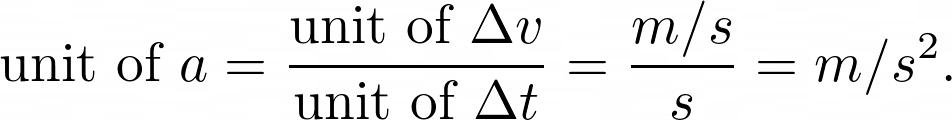
Similarly, we can use the equation involving b, and obtain its units. Let us use the equation we obtained earlier -

Using this, we can write -

which means,

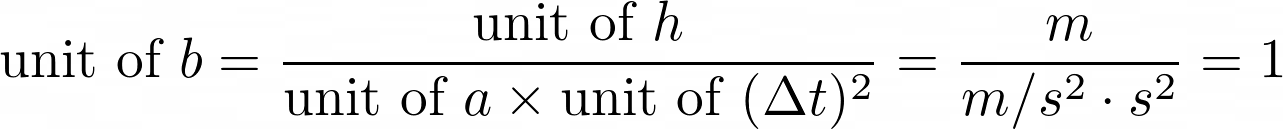
We see that the unit of b turns out to 1, and doesn’t correspond to any physical quantity like length, mass or time. This is understood as b not having any units at all. Such unit-less quantities are just pure numbers, for e.g. π that comes in the formula of the area of a circle.

Therefore, we conclude that the units of a and ab are same, because b is unit-less. So, the value of the constant product ab with its proper unit is 5 m/s².

This is essentially the whole description of Galileo’s law. He obtained a very simple formula that correctly predicts the motion of falling objects. Even though it is a huge achievement, it is fair to say that he could not have possibly studied allthe objects in the world, and maybe the law fails under some special circumstances.

Let us now try to think of some of the problems that he might have ignored -

• Friction caused due to air — It’s quite remarkable that Galileo correctly identified that if the objects are much denser than air, the effects due to air friction can be safely ignored. This is a big deal because, one of the hardest things to do in science is to isolate the non-essential aspects of a problem. Now, this doesn’t seem to be significant for heavy objects, but if one is interested in the behaviour of lighter objects in the presence of air, it should be possible to modify the law accordingly (a discussion reserved for a future article).
• Objects falling from greater heights — This problem is a bit tricky. We are trying to argue that, maybe the formula given by Galileo should depend on the heights from which the objects are dropped. Now, there is no obvious reason for this to happen, but we think that he may not have tested the formula for such cases. In Galileo’s time this wasn’t a deal breaker, but in modern sciences, we believe that

If a theory has not been tested under all conditions, then it is almost certain to fail under some of those conditions

This statement is the key to make progress in sciences. We should always allow the possibility for a great theory to be spectacularly wrong!

Now, as listed above, we have two possible problems in Galileo’s theory that we could study. Let us pick the second one and see how far we can go.

For a quick demonstration, let us imagine that we drop an object from a height of 800 meters. Such great heights must have been inaccessible to Galileo for doing controlled experiments, and that is the most likely explanation as to why he did not consider extending the law for greater heights.

We use Galileo’s formula to obtain the time required by the object to hit the ground as,

So, according to Galileo, an object dropped from 800 meters, should take approximately 12.649 seconds to hit the ground. But, if we actually measure the time using a clock, we would obtain the value to be approximately, 12.651 seconds, which is off by about 0.01%. No wonder, Galileo did not care about an improvement — the law really is quite accurate. This discrepancy between the theory and actual measurements will get bigger (as we will see later) if we drop the objects from even greater heights, in which case we surely would require a better theory.

Improving The Galileo’s Law of Falling Objects

So we now understand how Galileo obtained his law of falling objects — by repeated experimentation. If we hope to make any correction to the law, we must do the same.

First, we would like to have a “corrected” version of the formula as a hypothesis, which could be tested for validity. But, how do we get one ?

One of the coolest ideas is physics is that — we can only add (or subtract) quantities which have same units. We obviously cannot add speed of a moving object to its distance or time or anything else — it would make no sense at all. On the other hand, a multiplication (or division) of two quantities with different units is allowed. For example, we divide the distance travelled by an object by the time it takes to cover that distance, to obtain its speed. We are going to use this idea to come up with an improvement.

Let us go back to the original formula given by Galileo -

This can also be written as

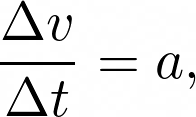
which basically means that change in speed of the falling object over an interval of time is always the same constant, a. This is just another way of stating the same Galileo’s law.

Now, the new formula must have a correction term that quantifies the dependence on the height of the falling object. Possibly the simplest way to include this effect is to have a correction term like -

Again, this might seem totally random, and we do not know as of now, if this would work, as there are literally infinite number of ways to include a correction. So, how do we know that we have chosen the right one? The answer is still the same — we shall go along with it unless it contradicts an experimental observation. If it doesn’t work, we would have to make another educated guess, based on experimental data. This is the reason it takes decades for a good theory to be developed — a lot of guesswork is needed before we find the right track.

As discussed earlier, this new correction term must have the same units as that of a, so that we can add this term to the old formula, without violating the rules of addition (subtraction) of physical quantities.

Since this equation has a proportionality, we need yet another constant to fix it, by writing it as

Let us now explore what kind of a quantity is c. In terms of units, we can write this equations as,

which means,

From this, we can easily see that,

It seems like, c is composed of a ratio of two other constants, whose units are m/s²in the numerator and m in the denominator. Lucky for us, we already have a constant lying around, with the units of m/s² — the constant a, and maybe we could try to use it for the numerator. But, we also need a constant in the denominator. So far, we have not seen any constant in the theory with units of length (meter).

Is it possible to imagine a “natural” choice for such a constant?

Since this constant has to be included artificially, there is no obvious way to obtain it from the physical observations. So, we have to use mathematical logic, as much as possible, to come up with an acceptable solution. To do that, we write down the basic requirements that this constant must fulfil -

• Since this constant is sitting in the denominator of the correction term, its value should not be too small, otherwise the correction term will become too big. Also, the units of the constant is meter, which should be associated with some kind of length or height. We know that Galileo’s law works very well for heights up to a few hundred meters, so we do not require a significantly large correction for heights of that range. This suggests that the value of this new constant must be beyond the few hundred meters mark, so that we do not spoil Galileo’s results. A reasonable guess could be something like 1000 or 2000 meters, which could be associated with some nearby tall structure.
• There doesn’t seem to be a connection between falling objects from a certain height and this mysterious tall structure with a height of around 1000 meters. Moreover, this mysterious structure should remain unchanged for allkinds of objects dropped from all kinds of heights, because we want this height to represent some sort of universal constant. Since we are introducing artificial corrections, we may never know the true connection between the falling objects and this tall structure, and only a deeper law of nature could reveal it. So, for now, we cannot hope to do anything about it, and we just follow the mathematical logic. (A few decades later, Issac Newton is going to resolve the mystery).
• It is not hard to imagine that even if this strategy works, the new law would only be good for a couple of thousand meters, and another revision might be needed if we go beyond that mark. It makes a lot of sense, to choose some kind of an “ultimate height” that would keep the law working for almost all cases imaginable. Now, the tallest structure on earth above sea level is the Mount Everest (approximately 8850 meters). Surely, a law that works well up to that height should be pretty good for a foreseeable future. For someone living in the 17th century, this would be more than good enough for most practical purposes and we could stop here.
• We may push our limits of imagination a bit further, and allow the possibility that there might be a scenario where some objects might be dropped from even greater heights, say from the sky. Now that the Mount Everest becomes insignificant in comparison, we need a new “ultimate height”. Since there is nothing else around, the only giant structure we have is the earth itself. Therefore, we must choose the size of the earth as the “ultimate height”. We have two quantities associated with the earth with units of length — the circumference and the radius. At this moment, there is no way to find which choice will work better, if at all. Here we choose the radius of the earth as the value of the new constant, and see how far we can go. If it fails, we can make the other choice and repeat.

With all this in mind, we may finally write the constant c with a in the numerator and the radius of earth in the denominator as,

where a is the same constant from Galileo’s old formula, and Rₑ is the radius of the earth. So, the full correction terms becomes -

There is one important point that we have to understand here — the whole idea is to improve the Galileo’s law, but we cannot deny that the old law is pretty good for a lot of cases, and it doesn’t really need much tinkering. So, it is very important that introduction of any new correction like this, must not affect the old formula in any drastic way.

One easy way to ensure that, is to include a “strength factor”, whose purpose is to control the “strength” of the new corrections coming into picture. The value of this factor, as always, can be determined by doing a lot of experiments. Once its value is determined, we would have obtained the final version of the improved formula.

With all this in mind, we add the correction term to Galileo’s original formula to get

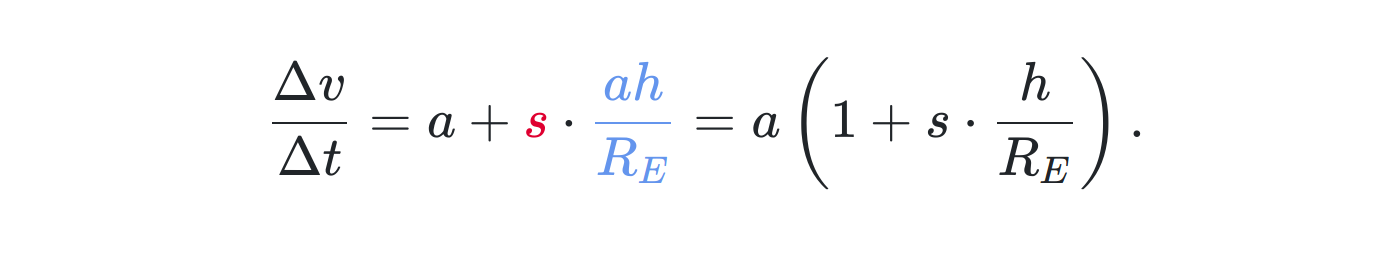
where s is the “strength factor”. It is intuitively clear that if we do not want any new effects, then the value of s should be zero, in which case we get back the old formula, as expected.

Let us call this formula, the “Improved law of falling objects”. Just for comparison, the old formula looked like this -

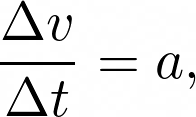
If we look carefully at the new formula, we observe that, we have effectively replaced the constant a on the right hand side, in the old formula with a new term given by,

That’s all we have changed, and maybe we could say that we have “effectively” corrected the value of the constant a, instead of correcting the whole theory. This means, if we call the effective value of a as a’, then the improved law of falling objects can simply be written as

This formula looks almost like the old formula, and therefore, we can borrow most of the results obtained earlier, with the understanding that all occurrences of a must be replaced by a’.

Let us rewrite the old formula of the height of the falling object, obtained earlier as

Using this result, we can easily obtain the time interval during which the object falls through height h as -

Now, we are ready to see the improved formula of height by replacing a by a’, to get -

and the time duration becomes -

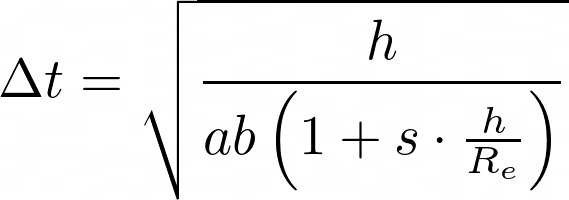
(A better and technically more correct method to obtain this result involves quite a bit of calculus, and is beyond the scope of this article)

Now, we ask the inevitable question — Why should we trust all this? We have made quite a few assumptions for getting this new formula, and to be honest, it certainly doesn’t “look” as nice as the old Galileo’s law. So, unless this new formula is correct, or at least offers any serious advantage over the old one, it may be foolish to stick with it.

On The Verge of A Breakthrough

Now we have developed a hypothesis, and all that remains to be done is — to drop some objects from a set of different known heights and measure the time taken to hit the ground in each case. It has to be kept in mind that the chosen heights must be much greater than what Galileo used to validate his old law.

The known quantities in the new formula are:

• The approximate average value of the radius of earth is, Rₑ= 6400000 m.
• The approximate value of the constant ab, as obtained by Galileo, is ab = 5 m/s².

By making a lot of measurements of the time taken for the objects to the hit the ground (this could take many years of experimentation!), we would find that the average value of the “strength factor” that gives the correct values of respective heights, comes out to be,

The following table summaries the results we would obtain -

(*These values are obtained using the “correct” theory of gravitation by Issac Newton)

We might consider this result as a significant achievement. Not only have we been able to identify a problem in the great old Galileo’s law, we have also been able to fix it, (arguably) without introducing too many new ideas.

In a few decades, Issac Newton would come up with an explanation as to why the value of s is equal to -2, by revealing an even deeper law of nature — the law of universal gravitation, and we would finally have a genuine scientific breakthrough.

Originally published at https://physicsgarage.com on May 18, 2020.